total mechanical energy problem solving

StickMan Physics

Animated Physics Lessons

Mechanical Energy Problem Solutions

Mechanical energy problems and solutions.

See examples of mechanical energy problems involving kinetic energy, potential energy, and the conservation of energy. Check your work with ours.

1. How much gravitational potential energy do you have when you lift a 15 N object 10 meters off the ground?

2. How much gravitational potential energy is in a 20 kg mass when 0.6 meters above the ground?

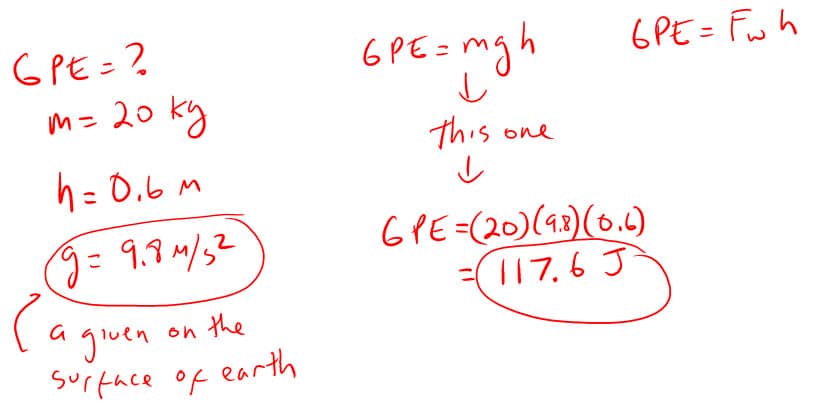
3. How much gravitational potential energy does a 35 kg boulder have when 30 meters off the ground?

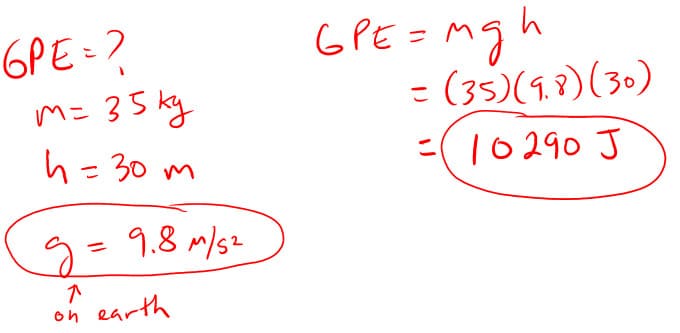
4. How many times greater is an objects potential energy when three times higher?

If you need help on ratio problems click the link below:

Rule of Ones: analyzing equations to determine how other variables change

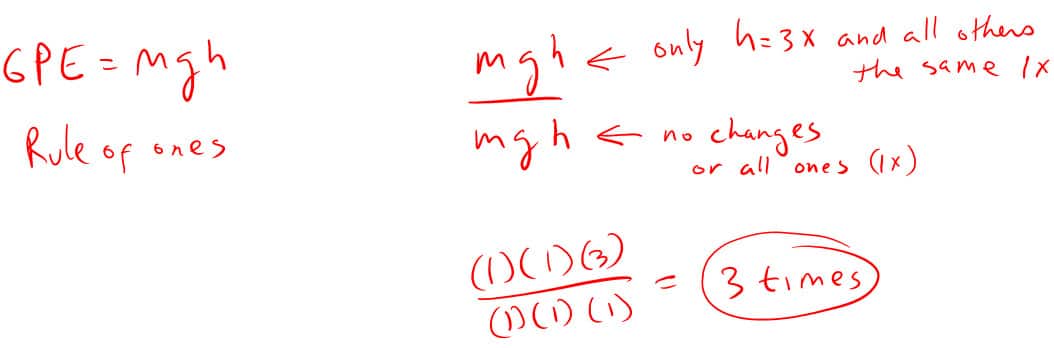
5.  How much kinetic energy does a 0.15 kg ball thrown at 24 m/s have?

6.  How many times greater is the kinetic energy of a ball that is going five times faster?

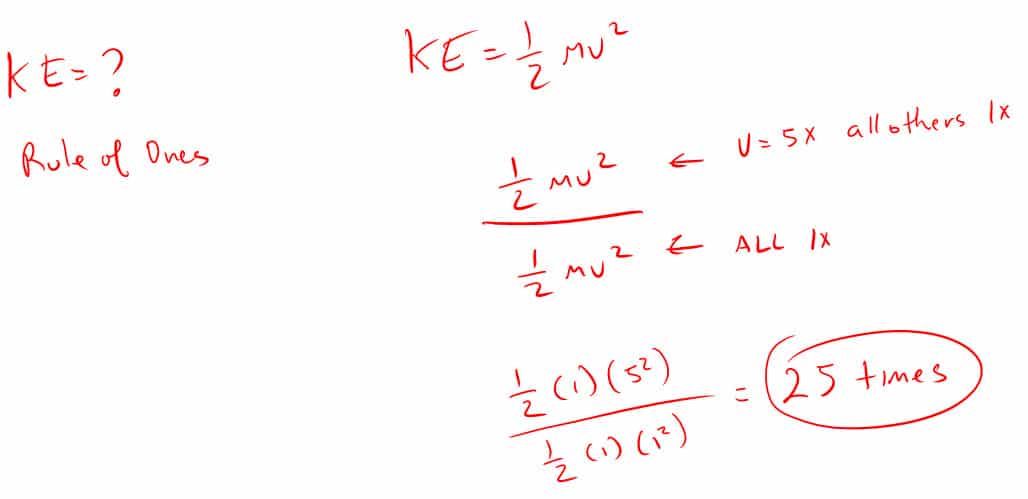
7.  How much kinetic energy does a 1.2 kg ball have the moment it hits the ground 3.5 meters below when it starts from rest?

I cancelled out the initial kinetic energy because:

• KE i = ½ mv f 2
• KE i = (½)(3.5)(0 2 ) = 0 J

I cancelled out the final potential energy because:

• PE f = mgh f
• PE f = (3.5)(9.8)(0) = 0 J

8.  How fast is a 1.2 kg ball traveling the moment it hits the ground 3.5 meters below when it starts from rest?

(Note: In many of these problems I could cancel out mass but did not since it was provided)

Since I did not cancel out mass I could answer the following questions if asked:

• How much mechanical energy did you have at the beginning? (41.6 J)
• How much kinetic energy did you have at the beginning? (0 J)
• How much potential energy did you have at the beginning? (41.6 J)
• How much potential energy do you have at the end? (0 J)

If I cancelled out mass in my work it would not show the actual initial potential energy since PE i = mgh and not just gh.

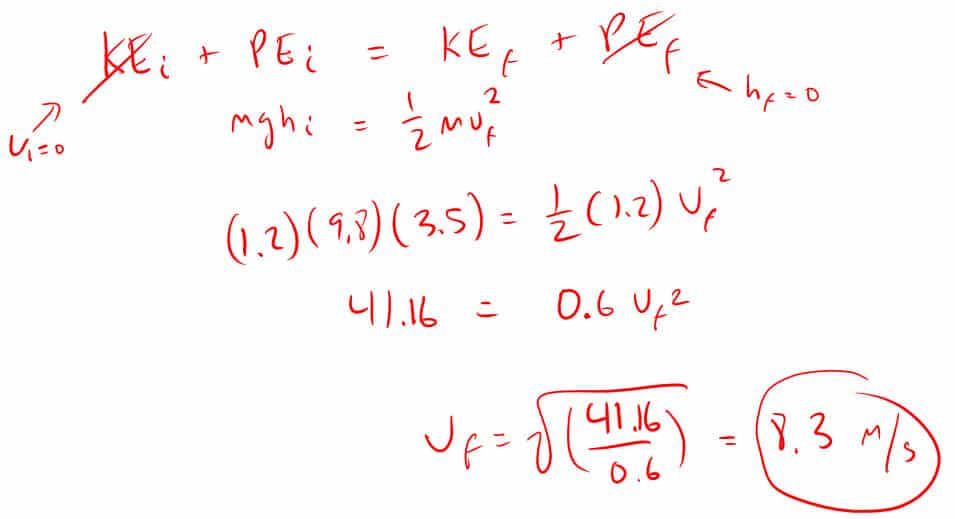
9.  A 3.5 kg ball fell from a height of 12 meters.  How fast is it traveling when its still 5 meters off the ground?

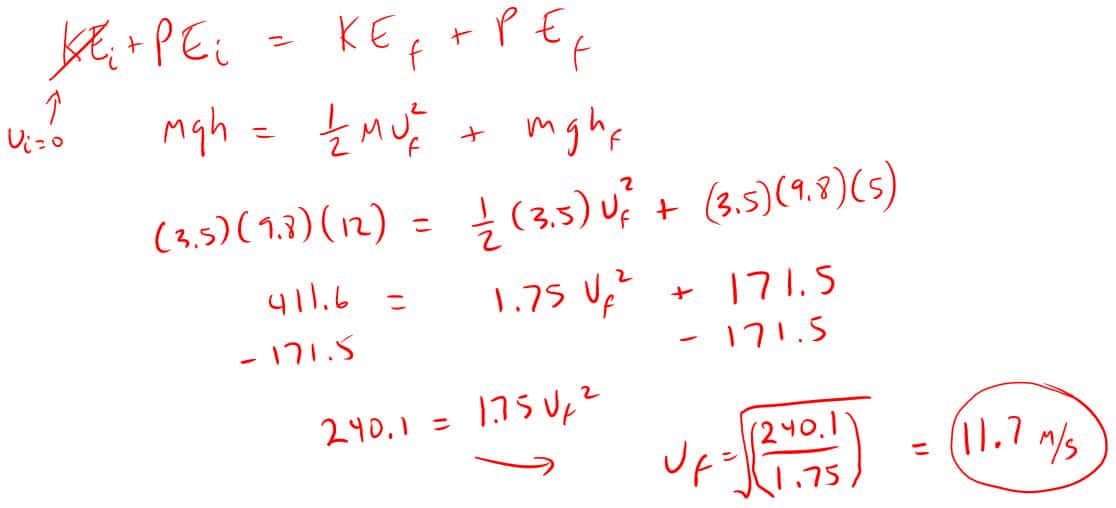
10. An 85kg roller coaster cart is traveling 4 m/s at the top of a hill 50 meters off the ground.  How fast is it traveling at top of a second hill 20 meters off the ground?

• Back to the Mechanical Energy Page
• Back to the Main Work, Power, Mechanical Energy, and Simple Machines Page
• Back to the  Stickman Physics Home Page
• For video tutorials and other physics resources check out  HoldensClass.com
• Find many of your animation resources in one place at the  StickMan Physics Gallery
• Equation Sheet

Terms and Conditions - Privacy Policy

Gurumuda Networks

Mechanical energy – problems and solutions

The work-mechanical energy principle

1. The coefficient of the kinetic friction between block and floor (μ k ) is 0.5. What is the displacement of an object (s)? Acceleration due to gravity is 10 m/s 2 .

The coefficient of the kinetic friction (μ k ) = 0.5

Mass of block (m) = 4 kg

Acceleration due to gravity (g) is 10 m/s 2

Weight of block (w) = m g = (4)(10) = 40 Newton

If block on the horizontal plane then the normal force (N) =weight (w) = 40 Newton.

If block on the horizontal plane then the normal force (N) = weight (w) = 40 Newton.

Initial velocity (v 1 ) = 5 m/s

Final velocity (v 2 ) = 0 m/s

Wanted : displacement of object (d) ?

The work-mechanical energy principle states that work (W) done by the nonconservative force is the same as the change of the mechanical energy of an object. The change of mechanical energy = the final mechanical energy – the initial mechanical energy.

The kinetic friction force is one of the nonconservative forces and the only one nonconservative force that acts on the block.

f k d = ME 2 – ME 1

Work done by the kinetic friction force :

W = f k d = (μ k )(N)(d) = (0.5)(40)(d) = 20 d

The change of the mechanical energy :

ΔME = ME 2 – ME 1 = (KE + PE) 2 – (KE + PE) 1

Object moves along the horizontal plane and no change of height (Δh = 0) so there is no change of the gravitational potential energy (ΔPE = PE 2 – PE 1 = 0). Thus the change of the mechanical energy just involves the change of the kinetic energy .

ΔME = KE 2 – KE 1 = ½ m v 2 2 – ½ m v 1 2 = ½ m (v 2 2 – v 1 2 )

Δ M E = ½ (4)(0 2 – 5 2 ) = (2)(25) = 50

Displacement of block :

s = 50 / 20

s = 2.5 meters

The principle of conservation of mechanical energy

2. Object A and object B have the same mass. Object A free fall from a height of h meters and object B free fall from a height of 2h meters. If object A hits the ground at v m/s, then what is the kinetic energy of the object B when it hits the ground.

The final velocity of object B when free fall from a height of 2h :

v 2 = 2 g (2h) = 4 g h

The kinetic energy of object B :

KE B = ½ m v 2 = ½ m (4 g h) = 2 m g h —– equation 1

The initial mechanical energy of object B = the gravitational potential energy = m g h.

The final mechanical energy of object B = the kinetic energy = ½ m v 2 .

The principle of conservation of mechanical energy :

m g h = ½ m v 2

Because m g h = ½ m v 2 then we can change m g h in equation 1 with ½ m v 2 .

The kinetic energy of object B = 2 m g h = 2(½ m v 2 ) = m v 2

3. An object free fall from a height of 20 meters. Acceleration due to gravity is 10 m/s. What is the velocity of object 15 meters above the ground?

The final mechanical energy = the initial mechanical energy

The kinetic energy at point 2 = the change of the gravitational potential energy as far as 5 meters.

4. A block is released from the top of the smooth inclined plane. What is the velocity of the block when hits the ground?

The final mechanical energy = the kinetic energy = 1/2 m v 2

The principle of conservation of mechanical energy, states that the initial mechanical energy = the final mechanical energy.

ME o = ME t

50 m = 1/2 m v 2

50 = 1/2 v 2

2 (50) = v 2

A block with mass of m-kg released from a height of h meters above the ground, as shown in figure below. Determine the ratio of the potential energy to the kinetic energy (KE) at point M.

PE M = m g (0.3 h)

The kinetic energy at point m = the change of the gravitational potential energy as far as h-0.3h = 0.7 h

KE M = PE = m g (0.7 h)

The ratio of the gravitational potential energy to the kinetic energy at point M :

PE M : KE M

m g (0.3 h) : m g (0.7 h)

6. If PE Q and KE Q have the potential energy and the kinetic energy at point Q (g = 10 m/s 2 ), then PE Q : KE Q =…

PE Q = m g h = (m)(10)(1.8) = 18 meters

The kinetic energy at point Q = the change of the gravitational potential energy as far as 5-1.8 = 3.2 meters

KE Q = PE = m g h = m (10)(3.2) = 32 m

The ratio of the gravitational potential energy to the kinetic energy at point Q :

PE Q : KE Q

18 m : 32 m

• What is mechanical energy? Answer : Mechanical energy is the sum of potential energy and kinetic energy in a system. It represents the total amount of energy associated with the motion and position of an object.
• How is kinetic energy different from potential energy? Answer : Kinetic energy is the energy of motion, while potential energy is the energy stored due to an object’s position or configuration. For example, a moving car has kinetic energy, while a stretched spring or an object at height in a gravitational field has potential energy.
• Is mechanical energy always conserved? Answer : In an ideal, closed system with no external forces, mechanical energy is conserved. However, in real-world situations, other forms of energy like thermal or sound energy can come into play due to friction or other non-conservative forces, leading to a decrease in total mechanical energy.
• How does the conservation of mechanical energy help in solving problems? Answer : When mechanical energy is conserved in a system (no non-conservative forces like friction), the total energy at the beginning is equal to the total energy at the end. This principle allows us to relate the potential and kinetic energies at different points in time, simplifying the problem-solving process.
• What happens to the mechanical energy of a ball when it’s thrown upwards and comes to a momentary stop before descending? Answer : As the ball rises, its kinetic energy decreases while its potential energy increases. At the peak, all its mechanical energy is potential energy. As it descends, this process reverses. In an ideal scenario without air resistance, the ball’s total mechanical energy remains constant throughout its journey.
• Can an object have both kinetic and potential energy at the same time? Answer : Yes, an object can possess both forms of energy simultaneously. For instance, a pendulum at the midpoint of its swing has kinetic energy due to its motion and potential energy due to its height above its lowest point.
• How does a roller coaster demonstrate the conservation of mechanical energy? Answer : A roller coaster starts with potential energy at its highest point. As it descends, this potential energy converts to kinetic energy, making the coaster speed up. As it climbs again, the kinetic energy reduces while potential energy increases. Ignoring friction and air resistance, the total mechanical energy remains nearly constant.
• What factors can lead to a loss of mechanical energy in real-world systems? Answer : Friction, air resistance, and sound generation are some factors that can lead to energy losses in real-world systems. The energy isn’t destroyed (due to the law of conservation of energy) but is transformed into other forms like heat or sound.
• What is the relationship between work and mechanical energy? Answer : Work done on a system by external forces can lead to a change in the system’s mechanical energy. In mathematical terms, work is equal to the change in mechanical energy.

If gravitational potential energy is relative to a reference point, how can we consistently measure changes in it? Answer : While the absolute value of gravitational potential energy is indeed dependent on the choice of reference point, changes in potential energy (like when an object is raised or lowered) are consistent regardless of the reference. Thus, we often focus on changes in potential energy rather than absolute values.

Share this:

Discover more from physics.

Subscribe now to keep reading and get access to the full archive.

Type your email…

Continue reading

You must be logged in to post a comment.

7.6 Conservation of Energy

Learning objectives.

By the end of this section, you will be able to:

• Explain the law of the conservation of energy.
• Describe some of the many forms of energy.
• Define efficiency of an energy conversion process as the fraction left as useful energy or work, rather than being transformed, for example, into thermal energy.

Law of Conservation of Energy

Energy, as we have noted, is conserved, making it one of the most important physical quantities in nature. The law of conservation of energy can be stated as follows:

Total energy is constant in any process. It may change in form or be transferred from one system to another, but the total remains the same.

We have explored some forms of energy and some ways it can be transferred from one system to another. This exploration led to the definition of two major types of energy—mechanical energy KE + PE KE + PE and energy transferred via work done by nonconservative forces ( W nc ) ( W nc ) . But energy takes many other forms, manifesting itself in many different ways, and we need to be able to deal with all of these before we can write an equation for the above general statement of the conservation of energy.

Other Forms of Energy than Mechanical Energy

At this point, we deal with all other forms of energy by lumping them into a single group called other energy ( OE OE ). Then we can state the conservation of energy in equation form as

All types of energy and work can be included in this very general statement of conservation of energy. Kinetic energy is KE KE , work done by a conservative force is represented by PE PE , work done by nonconservative forces is W nc W nc , and all other energies are included as OE OE . This equation applies to all previous examples; in those situations OE OE was constant, and so it subtracted out and was not directly considered.

Making Connections: Usefulness of the Energy Conservation Principle

The fact that energy is conserved and has many forms makes it very important. You will find that energy is discussed in many contexts, because it is involved in all processes. It will also become apparent that many situations are best understood in terms of energy and that problems are often most easily conceptualized and solved by considering energy.

When does OE OE play a role? One example occurs when a person eats. Food is oxidized with the release of carbon dioxide, water, and energy. Some of this chemical energy is converted to kinetic energy when the person moves, to potential energy when the person changes altitude, and to thermal energy (another form of OE OE ).

Some of the Many Forms of Energy

What are some other forms of energy? You can probably name a number of forms of energy not yet discussed. Many of these will be covered in later chapters, but let us detail a few here. Electrical energy is a common form that is converted to many other forms and does work in a wide range of practical situations. Fuels, such as gasoline and food, carry chemical energy that can be transferred to a system through oxidation. Chemical fuel can also produce electrical energy, such as in batteries. Batteries can in turn produce light, which is a very pure form of energy. Most energy sources on Earth are in fact stored energy from the energy we receive from the Sun. We sometimes refer to this as radiant energy , or electromagnetic radiation, which includes visible light, infrared, and ultraviolet radiation. Nuclear energy comes from processes that convert measurable amounts of mass into energy. Nuclear energy is transformed into the energy of sunlight, into electrical energy in power plants, and into the energy of the heat transfer and blast in weapons. Atoms and molecules inside all objects are in random motion. This internal mechanical energy from the random motions is called thermal energy , because it is related to the temperature of the object. These and all other forms of energy can be converted into one another and can do work.

Table 7.1 gives the amount of energy stored, used, or released from various objects and in various phenomena. The range of energies and the variety of types and situations is impressive.

Problem-Solving Strategies for Energy

You will find the following problem-solving strategies useful whenever you deal with energy. The strategies help in organizing and reinforcing energy concepts. In fact, they are used in the examples presented in this chapter. The familiar general problem-solving strategies presented earlier—involving identifying physical principles, knowns, and unknowns, checking units, and so on—continue to be relevant here.

Step 1. Determine the system of interest and identify what information is given and what quantity is to be calculated. A sketch will help.

Step 2. Examine all the forces involved and determine whether you know or are given the potential energy from the work done by the forces. Then use step 3 or step 4.

Step 3. If you know the potential energies for the forces that enter into the problem, then forces are all conservative, and you can apply conservation of mechanical energy simply in terms of potential and kinetic energy. The equation expressing conservation of energy is

Step 4. If you know the potential energy for only some of the forces, possibly because some of them are nonconservative and do not have a potential energy, or if there are other energies that are not easily treated in terms of force and work, then the conservation of energy law in its most general form must be used.

In most problems, one or more of the terms is zero, simplifying its solution. Do not calculate W c W c , the work done by conservative forces; it is already incorporated in the PE PE terms.

Step 5. You have already identified the types of work and energy involved (in step 2). Before solving for the unknown, eliminate terms wherever possible to simplify the algebra. For example, choose h = 0 h = 0 at either the initial or final point, so that PE g PE g is zero there. Then solve for the unknown in the customary manner.

Step 6. Check the answer to see if it is reasonable . Once you have solved a problem, reexamine the forms of work and energy to see if you have set up the conservation of energy equation correctly. For example, work done against friction should be negative, potential energy at the bottom of a hill should be less than that at the top, and so on. Also check to see that the numerical value obtained is reasonable. For example, the final speed of a skateboarder who coasts down a 3-m-high ramp could reasonably be 20 km/h, but not 80 km/h.

Transformation of Energy

The transformation of energy from one form into others is happening all the time. The chemical energy in food is converted into thermal energy through metabolism; light energy is converted into chemical energy through photosynthesis. In a larger example, the chemical energy contained in coal is converted into thermal energy as it burns to turn water into steam in a boiler. This thermal energy in the steam in turn is converted to mechanical energy as it spins a turbine, which is connected to a generator to produce electrical energy. (In all of these examples, not all of the initial energy is converted into the forms mentioned. This important point is discussed later in this section.)

Another example of energy conversion occurs in a solar cell. Sunlight impinging on a solar cell (see Figure 7.19 ) produces electricity, which in turn can be used to run an electric motor. Energy is converted from the primary source of solar energy into electrical energy and then into mechanical energy.

Even though energy is conserved in an energy conversion process, the output of useful energy or work will be less than the energy input. The efficiency Eff Eff of an energy conversion process is defined as

Table 7.2 lists some efficiencies of mechanical devices and human activities. In a coal-fired power plant, for example, about 40% of the chemical energy in the coal becomes useful electrical energy. The other 60% transforms into other (perhaps less useful) energy forms, such as thermal energy, which is then released to the environment through combustion gases and cooling towers.

PhET Explorations

Masses and springs.

A realistic mass and spring laboratory. Hang masses from springs and adjust the spring stiffness and damping. You can even slow time. Transport the lab to different planets. A chart shows the kinetic, potential, and thermal energies for each spring.

• 1 Representative values

As an Amazon Associate we earn from qualifying purchases.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute OpenStax.

Access for free at https://openstax.org/books/college-physics-2e/pages/1-introduction-to-science-and-the-realm-of-physics-physical-quantities-and-units

• Authors: Paul Peter Urone, Roger Hinrichs
• Publisher/website: OpenStax
• Book title: College Physics 2e
• Publication date: Jul 13, 2022
• Location: Houston, Texas
• Book URL: https://openstax.org/books/college-physics-2e/pages/1-introduction-to-science-and-the-realm-of-physics-physical-quantities-and-units
• Section URL: https://openstax.org/books/college-physics-2e/pages/7-6-conservation-of-energy

© Jan 19, 2024 OpenStax. Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License . The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

• Total mechanical energy is just the kinetic plus potential energy.
• As long as there are no outside forces unaccounted for, we know that the totals before and after will be equal.

E k + E p = E k ’ + E p ’

Note: the little ’ just means “after”.

We usually call this the Law of Conservation of Energy . It grows out of the Laws of Thermodynamics .

First Law of Thermodynamics

“Energy can not be created or destroyed, only changed from one form to another.”

• This just basically means that if one thing loses energy, something else must be gaining energy. The opposite is also true.
• In an ideal situation this transfer of energy would be perfect and complete, but when was the last time you remember our universe being perfect…? This leads us to the second law …

Second Law of Thermodynamics

“In any energy conversion, there will always be some waste energy released as heat into the surrounding environment.”

In the second law we recognize that the total energy is constant, it’s just that some of the energy is released as unusable heat.

• This energy naturally flows from a hotter source to the cooler environment.

In most of the work we do, we assume that we are living in a “perfect” universe.

• This means that for the most part we will obey the first law, but ignore the second.
• There will be some situations when we give you enough information to use the second law, but we will be pretty specific about telling you.
• Most of the time we will say something about the friction involved, since this is the most common source for heat loss in your problems

Example 1 : A person is sitting on a toboggan at the top of a 23.7m tall hill. If the person and toboggan have a total mass of 37.3 kg determine how fast they will be going when they reach the bottom of the hill. Assume there is no friction.

At the top of the hill the person isn’t moving, so E k will be zero. At the bottom of the hill the E p will be zero. E k + E p = E k ’ + E p ’ ½ mv 2 + mgh = ½ mv’ 2 + mgh’ 0 + (37.3kg) (9.81m/s 2 ) (23.7m) = ½ (37.3kg) v’ 2 + 0 8.67e3 J = 18.7 v’ 2 v’ = 21.6 m/s

Notice how in this example all of the potential energy the object had at the top of the hill has been turned completely into kinetic energy at the bottom.

• It’s also possible to analyze how the potential energy steadily changes into kinetic energy during a fall…

Example 2 : Wile E. Coyote is trying to drop a boulder off a cliff to hit the Roadrunner eating a bowl of birdseed. He wants to know the speed of the boulder at various points. He supplies you with the following blueprint…

The Coyote wants you to determine the velocity of the boulder at several different heights above the ground, assuming no air resistance…

a) 45 m b) 30 m c) 10 m d) 0 m a) Well, this one ain’t so tough! Since it’s sitting at the top of the cliff, its velocity is 0 m/s. It might be handy at this point to calculate how much E p the boulder has. E p = mgh = 200kg (9.81m/s 2 ) (45m) = 88290 J = 8.8e4 J TOTAL ENERGY = 8.8e4 J

b) First, ask yourself how much E p the boulder still has at 30m above the ground.

E p = mgh = 200kg (9.81m/s 2 ) (30m) = 58860 J = 5.9e4 J

That means that 8.8e4 J - 5.9e4 J = 2.9e4 J is missing, right?

Wrong! According to the conservation of energy, that energy must now be kinetic !

TOTAL ENERGY = 8.8e4 J

c) Again, calculate how much E p you have at this new height of 10 m…

E p = mgh = 200kg (9.81m/s2) (10m) = 19620 J = 2.0e4 J

That means that I have changed 6.9e4 J of energy into other forms… we’ll assume it all changed into kinetic energy.

d) By the time the boulder has reached the ground, all of its potential energy is gone (it’s zero metres above the ground!). We all know that when it actually hits the ground it will come to rest, but we are concerned with how fast it’s going when it is right at ground level but hasn’t actually touched the ground yet. We can assume that all of the potential energy the boulder had at the top is now kinetic energy at the bottom…

You could be finding the same answers based on kinematics formulas from Physics 20.

• In fact, you’ll find that conservation of energy gives you new ways to do many problems that you did with kinematics formulas…

Forgot password? New user? Sign up

Existing user? Log in

Conservation of Energy

Already have an account? Log in here.

• Shabarish Ch
• Kishore S. Shenoy
• Aakash Khandelwal
• Shubhang Sharma
• Josh Silverman

Some of the great tools in physics are so-called "conservation laws" that buttress the laws of motion with certain quantities that remain the same throughout time. Among these great laws is the conservation of energy which states that while energy can change forms, it cannot be created or destroyed.

Here we'll explore the partition between kinetic energy and potential energy, and how energy can in some sense replace forces in our calculations. We conclude with the introduction of formal methods of physics that analyze the dynamic behavior of systems solely in terms of energy, thus replacing the necessity of having to analyze forces at all. Such methods play a dominant role in systems of condensed matter, quantum field theory, and other problems far beyond elementary classical mechanics.

The Conservation of Kinetic Energy

Work-energy theorem, work with potential energy, conservation of kinetic and potential energies, conservation of energy in general, hamiltonians, lagrangians, and noether's theorem, additional conservation of energy problems solving.

For a person riding a bicycle, their kinetic energy \(E\) is equal to the amount of heat that would be dissipated from the moment they pull the brake to the moment they come to rest. If they don't skid, most of this heat will go into heating the brake pad and the metal rim of the wheel. If they do skid, most of the heat will go to the rubber in the tire and to the road. Before they pull the break, this energy can be observed as the ongoing motion of the parts of the bicycle, i.e. the net forward movement of the bicycle and the spinning of the wheels.

There are other forms of energy that a particle can have such as gravitational potential energy, chemical potential energy, electrical potential energy, spring potential energy, et cetera, but the kinetic energy is the portion of an object's energy that is due explicitly to its ongoing motion. For a point particle of mass \(\delta m\), moving at speed \(v\), the kinetic energy is given by the formula \(\frac12 \delta mv^2\), and the kinetic energy of any extended object can be built up from this. If a moving object collides with another object, and there is no dissipation, (no heat is given off, no chemical bonds are rearranged, etc.) then the total kinetic energy of the objects after the collision must equal the kinetic energy of the moving object before the collision.

In Isaac Newton's Principia Mathematica , momentum is defined and is said to be conserved, but there is no mention of energy anywhere in his treatise. Newton was able to work out the elliptical orbits of planets without any reference to energy at all. However, if we start with the assumption that momentum is conserved, we can derive the conservation of kinetic energy, and thus obtain the structure that lay hidden from Newton in his own theory.

Colliding balls on a table Consider a ball of mass \( m_{1} \) moving with velocity \( v_{0} \) in the direction of a stationary ball of mass \( m_{2} \). Let the velocities of the balls after the collision be \( v_{a} \) and \( v_{b} ,\) respectively. Moreover, suppose the collision is elastic, i.e. the relative velocity of approach is equal and opposite to the relative velocity of separation, i.e. \(\dfrac{\Delta v_i}{\Delta v_f} = -1\). Therefore, along with the conservation of momentum, we have \[\begin{align} v_{0} &= v_{b} - v_{a} \\ \\ m_{1}v_{0} &= m_{1}v_{a} + m_{2}v_{b}. \end{align}\] Solving these two equations for \( v_a \) and \( v_b ,\) we find \[\begin{align} v_a &= \dfrac{m_1 - m_2}{m_1 + m_2}v_0 \\\\ v_b &= \dfrac{2m_1}{m_1 + m_2}v_0. \end{align}\] Let us now calculate the initial and final kinetic energies \(\big(\frac12 mv^2\) for each ball\(\big):\) \[\begin{align} \textrm{KE} _{i} &= \dfrac{1}{2}m_{1}v_{0}^2 \\\\ \textrm{KE} _{f} &= \dfrac{1}{2}m_{1}v_{a}^2 + \dfrac{1}{2}m_{2}v_{b}^2. \end{align}\] Using the values of \( v_a \) and \( v_b \) from above we find (after some tedious algebra) \[\textrm{KE} _{initial} = \textrm{KE} _{final} .\] Hence, the total kinetic energy is conserved in elastic collisions. What about during the collision? Imagine we have two identical balls speeding with equal but opposite velocities to a collision. For an instant, neither ball is moving. So where did the kinetic energy of the balls go during this instant? The collision of the balls is not instantaneous. For a short interval, the kinetic energy of the balls is stored as elastic potential energy, after which it is reallocated to restore the original kinetic energy of the balls. Elastic energy is the potential energy stored in a deformed body, as for example with the compression of an elastic spring. At all times, the combined kinetic and elastic potential energy of the balls is constant. For more information on elastic collisions, see Analyzing Elastic Collisions .

In the previous example, we saw that the conservation of energy falls out in elastic collisions. What about when an object is accelerated by a gravitational field? Without a detailed understanding of potential energy, can we identify quantities that exchange with kinetic energy? Below, we analyze an especially profitable example of a skier on a frictionless slope.

The inclined plane Under constant acceleration, an object travels a distance \(d\) in the time \(t = \sqrt{\frac{2d}{a}}\). For a skier on an inclined plane, this time is given by \(\sqrt{\frac{2d}{g\sin\theta}}\). Also, starting from rest at the top of the incline, the skier has speed \(v = at = g\sin\theta \sqrt{\frac{2d}{g\sin\theta}} = \sqrt{2g\sin\theta d}\) at the bottom of the incline. Consider the change in kinetic energy \(\frac12 mv^2\). At the top of the incline, this quantity is equal to zero, and at the bottom of the incline it's equal to \(mgd\sin\theta\). We notice that \(mgd\sin\theta\) is simply the strength of the gravitational force times the vertical distance through which the skier has descended. Further, we notice that the quantity \(\frac12 mv^2 + mg(h_0-h)\) is a constant throughout the course of the skier's motion, i.e. kinetic energy exchanges with the quantity \(mg\Delta h\). Evidently, by falling through the distance \(\Delta h\), the skier has picked up kinetic energy \(\text{KE} = mg\Delta h\). This suggests that \(mg\Delta h\) is equal to the gravitational potential energy of the skier, which can reallocate to the kinetic energy, based on the skier's vertical position.

Thus, we have stumbled upon a conservation relation between kinetic energy and the gravitational potential energy. As you might imagine, in this example, the conservation is imposed by the fact that the system follows Newton's second law \(F = \frac{dp}{dt}\).

In general, we'll see that energy conservation holds in countless other scenarios. Further, in all the centuries of careful experimentation, a violation has never been identified (in macroscopic systems). For this reason, the conservation of energy has been elevated to the status of a law, i.e. a principle that is expected to hold for all processes in the universe.

In plain English, work means to do something productive. In physics, work is said to be done if force acts to displace an object. For example, when you push a sled across the snow, or lift a bucket of water onto a ledge, or pump up the tire on your bicycle, you've done work. This idea is captured in the definition of work which is the applied force along the direction of displacement times the displacement. Mathematically, we can write

\[W=\int \vec F\cdot d\vec s = Fs\cos\theta, \]

where \(\theta\) is the angle between the force and displacement vectors.

As you might imagine, performing work on an object cannot only change its displacement but its kinetic energy as well. We return once more to the example of the skier on the slope to see how these ideas are related.

Work on the skier Recall our results from above regarding the skier on the slope. Now, consider the work \(F\cdot s\), where \(F\) is the force of gravity along the incline, and \(s\) is the distance traveled along the incline. With \(F = mg\sin\theta\) and \(s=d\), we find \(F\cdot s = mg\sin\theta d\). This is curious. In descending the slope, we showed that the skier gained an amount of kinetic energy \(mgd\sin\theta\) which is also equal to the product of the force that acted on the particle, and the distance over which the particle traveled. Moreover, if we were to pull the skier up the slope at constant velocity, he'd have gained potential energy in the amount \(mgd\sin\theta\). One might be tempted to hypothesize that work delivers energy to objects. Indeed, during his descent the gravitational field does work upon the skier to speed him toward Earth, and in pulling him up the hill, we do work against the gravitational field. Work against a field can always be recouped later and thus we say it is stored kinetic energy or, in other words, potential energy.

Mathematically, we've shown \(\vec{F}\cdot\vec{d} = \Delta \textrm{KE} +\Delta \textrm{PE}= \frac12mv_f^2-\frac12mv_i^2 + mg\left(h_f-h_i\right)\).

This suggests that the cumulative pull of the skier by gravity has given rise to the kinetic energy of the particle at the expense of his initial potential energy. Thus, we can either look at the exchange as work done on the skier by the gravitational field, or as a reallocation of potential energy to kinetic energy.

This relationship would be quite useful if it holds in general, but as yet, it is just a nice coincidence we've noticed in our calculation. Next we look to Newton's laws for a way to put it on firm ground.

Newton's laws: a basis for work According to the second law, we have the following relationship between changes in velocity and a net applied force (for simplicity we work in one dimension, though the result easily generalizes): \[\begin{align}F_\textrm{net}&=ma\\ &=m\frac{\Delta v}{\Delta t}.\end{align}\] Rearranging, we have \[\begin{align} F_\textrm{net} \Delta t &= m \Delta v \\ &= \Delta p.\end{align}\] Because \(\displaystyle\Delta x = v \Delta t\), we can write \(\displaystyle F_\textrm{net} \Delta x / v = m \Delta v\), or \[ F_\textrm{net} \Delta x = m v\Delta v. \] This relation shows that if the object is traveling with velocity \(v\) and it is pushed through some small distance \(\Delta x\) parallel to the force \(\displaystyle F_\textrm{net}\), it will pick up the additional velocity \(\displaystyle \Delta v = \frac{F_\textrm{net}\Delta x}{mv}\). In three dimensions, our result is \(\displaystyle \vec{F}_\textrm{net}\cdot\Delta\vec{x} = m\vec{v}\cdot\Delta\vec{v}\). This relation provides the basis for what we suspected above, that forces can do work to endow particles with kinetic energy. We can now exploit the relation to prove the work-kinetic energy theorem.

Relation between work and kinetic energy

Work-Kinetic Energy Theorem The net work (i.e. work minus work performed against any external fields) done by the force on an object is equal to its change in kinetic energy: \[\Delta \text{KE} = \int \vec F \cdot d\vec s.\]

Now think about the ways in which you can prove this theorem before you reveal the proof, try proving it yourself, and don't worry if you weren't able to crack it because most of the people can't do it in a single go.

Proof for Work-Energy Theorem

Proof 1 : If we add up all of the incremental pushes \(F_\textrm{net}\Delta s\) that the particle receives over the distance \(d\), we get \(F_\textrm{net}\sum \Delta s = F_\textrm{net}d\). However, we showed that \(F_\textrm{net}\Delta s = mv\Delta v\), so we have \(F_\textrm{net}d = m\sum v\Delta v\), a sum over the incremental increases in velocity. Let us now perform the sum of the \(mv\Delta v\). To start, when \(v\) is zero, \(mv\) is zero; at the end, it is equal to \(mv_f \). If we divide the increases up into \(n\) equal pieces, the velocity increases \(\Delta v\) are each given by \(\frac{1}{n}v_f\), which we can pull out of the sum so that the sum becomes \(\dfrac{v_f}{n}\sum v\). Suppose we divide the velocity increase over many moments so that \(n\) is large and the changes are small. Now, the sum of \(v\) from \(0\) to \(v_f\) in \(n\) equally sized chunks is simply \(v\) times the average value of \(v\) over the range: \[\sum\limits_{v=0}^{v_f} v= n\frac{v_f}{2}.\] Therefore, \(\sum mv\Delta v\) is equal to \[\dfrac{mv_f}{n}\sum\limits_{v=0}^{v_f}\Delta v = \frac{mv_f}{n}n\dfrac{v_f}{2} = \dfrac12 mv_f^2,\] which implies \(F_\textrm{net}d=\frac12 mv_f^2.\) This proves that if we act on an object of mass \(m\) with a force \(F_\textrm{net}\) over a distance \(d,\) it ends up with a kinetic energy \(\frac12 mv_f^2\), where the velocity \(v_f\) is given by \(\sqrt{2F_\textrm{net}d/m}\). \(_\square\) \[\] Proof 2 : We know \[W = \int \vec F \cdot d\vec s.\] Since \(\vec F =m \vec a=m\dfrac{d\vec v}{dt},\) it follows that \[\begin{align} W &= \int \vec F \cdot d\vec s \\ &=\int m\dfrac{d\vec v}{dt}\cdot d\vec s =\int\limits_{\vec {v_0}}^{\vec{v_f}}m\dfrac{d\vec s}{dt} d\vec v \\ &=m\int\limits_{\vec {v_0}}^{\vec{v_f}} \vec v \cdot d\vec v =\Delta \textrm{KE}, \end{align}\] which proves \(\Delta \textrm{KE} = W. \ _\square\)

Thus, a force acting on an object through a distance transfers energy to the object, and we call this quantity, \(W_\textrm{net} = \displaystyle\int \vec{F}\cdot d\vec{s}\), the work. In a frictionless system, the work is equal to the change in energy caused by the force:

\[W_\textrm{net} = \Delta \textrm{KE} + \Delta \textrm{PE}.\]

More generally, the work is equal to the change in energy plus whatever heat is dissipated to the environment.

Potential energy is a form of energy due to the configuration or position of an object in a field. It can be seen as a stored form on kinetic energy in that it can be converted to kinetic energy by allowing an object to relax its position or configuration in a field. For example, if we release an electric dipole previously restrained at an angle in an electric field, or drop a massive object in a gravitational field, potential energy is converted to movement through relaxation.

Dams contain massive amount of water stored at a considerable height. When this water is released, the gravitational potential energy is converted to kinetic energy and the water flows, which in turn spins the turbines to generate electricity which can be stored as electric potential energy in batteries. Thus the water which was stored at a height is used to generate electricity in a time of scarcity. Thus kinetic energy can be captured and stored in various forms of potential energy to be extracted later.

Gottfried Wilhelm Leibniz in 1676-1689 was the first to attempt a definition of kinetic energy, and noticed that for some mechanical systems it seemed to be conserved. But remarkably, it was a French mathematician, Emilie du Chatelet, who was the first to propose and derive the conservation of total kinetic and potential energy. She was aware of Isaac Newton's Principia Mathematica and undertook its translation into French, which she completed in 1749. During those years of translation, she included her derivation of the conservation law as a supplement to Newton's treatise. Soon afterwards, mathematicians Leonhard Euler and Joseph-Louis Lagrange went on to develop a mathematical formalism of classical mechanics, based on her works.

The Ball In this example, we see the interplay of kinetic and potential energies of a falling ball such that their sum is constant, when air resistance is ignored. Consider a ball of mass \( m \) falling from rest from a height \( H \) above the ground. Initially, since the ball is at rest its kinetic energy and potential energy, respectively, are \[\begin{array} &\textrm{KE}_i = 0, &\textrm{PE}_i = mgH. \end{array} \] Let \(v\) be the speed of the ball when it is at height \( h \) above the ground. From the second kinematic equation we have \( v^2 = 2g(H-h) \). Hence, the kinetic energy of the ball at height \( h \) is \[\textrm{KE}_h = \dfrac{1}{2}mv^2 = mg(H-h). \] From the expression of potential energy, the potential energy at height \( h \) is \[\textrm{PE}_h = mgh. \] Thus, the sum of energies is \(\textrm{KE}_h + \textrm{PE}_h = mgH \), which is the same as the initial sum of energies.

Spring With Oscillating Mass When an ideal spring is either compressed or stretched a distance \(x\) from the origin where the spring force \(=0\), the potential energy stored in the spring with a spring constant \(k\) \((\)which comes from using force to compress the spring against the spring force \(F=kx)\) is \[PE = \dfrac{1}{2}kx^2,\] while kinetic energy of the oscillating mass \(m\) moving at velocity \(v\) is \[KE = \dfrac{1}{2}mv^2,\] the sum of which is the total energy \(E\). \(\;\) Since total energy is conserved , \(E\) is a constant over time, so differentiating both sides by time \(t\), we have \[\dfrac { d }{ dt } E=0 =\dfrac {dx}{dt} \dfrac { d }{ dx } \left( \dfrac { 1 }{ 2 } k{ x }^{ 2 } \right) +\dfrac {dv}{dt} \dfrac { d }{ dv } \left( \dfrac { 1 }{ 2 } m{ v }^{ 2 } \right) \implies vkx+amv=0.\] Simplifying, we have \[ -kx = ma = F,\] which is Hooke's Law stating that the spring force exerted on mass \(m\) at any point is equal to \(-kx\), a linear function of the distance from the origin where the force \(= 0,\) in the direction towards the origin. This can be restated in terms of time \(t\) \[\dfrac { m }{ k } \dfrac { { d }^{ 2 }x }{ d{ t }^{ 2 } } +x =0,\] a \(2^{\text{nd}}\) order differential equation which has the solution, where \(b\) is a constant: \[x \left( t \right) =b\sin\left( \sqrt { \dfrac { k }{m } }\, t \right). \] Thus, the spring with oscillating mass has a period \(T,\) which is \[T=2\pi \sqrt { \dfrac { m }{ k } }. \]

The pendulum When a pendulum of massless arm length \(L\) with mass \(m\) at the end of it is at angle \(\theta\) from vertical, the gravitational potential energy is \[PE=mgh=mgL(1-\cos\theta), \] while the rotational kinetic energy of the mass \(m\) is \[KE=\dfrac { 1 }{ 2 } I{ { \omega }^{ 2 } }=\dfrac { 1 }{ 2 } m{ L }^{ 2 }{ \omega }^{ 2 },\] the sum of which is the total energy \(E\). \(\;\) Since total energy is conserved , \(E\) is a constant over time, so differentiating both sides with respect to time \(t\), we have \[\begin{align} \dfrac { d }{ dt } E&=0\\ \dfrac { d\theta }{ dt } \dfrac { d }{ d\theta } [mgL( 1-\cos\theta)] +\dfrac { d\omega }{ dt } \dfrac { d }{ d\omega } \left( \dfrac { 1 }{ 2 } m{ L }^{ 2 }{ \omega }^{ 2 } \right)&=0 \\ \dfrac { d\theta }{ dt } mgL\sin\theta +\dfrac { d\omega }{ dt } m{ L }^{ 2 }\omega &=0\\ \frac { d\theta }{ dt } mgL\sin\theta +\frac { { d }^{ 2 }\theta }{ d{ t }^{ 2 } } m{ L }^{ 2 }\frac { d\theta }{ dt }&=0 \\ \dfrac { L }{ g } \dfrac { { d }^{ 2 }\theta }{ d{ t }^{ 2 } } +\sin\theta &=0. \end{align}\] As an approximation for small \(\theta\), we have \[\dfrac { L }{ g } \dfrac { { d }^{ 2 }\theta }{ d{ t }^{ 2 } } +\theta =0,\] a \(2^{\text{nd}}\) order differential equation which has the solution, where \(b\) is a constant: \[\theta \left( t \right) =b\sin\left( \sqrt { \dfrac { g }{ L } }\, t \right). \] Thus, the simple pendulum, for small \(\theta\), has a period \(T\) which is dependent only on \(L\) and \(g:\) \[T=2\pi \sqrt { \dfrac { L }{ g } }. \] Or more generally, for small \(\theta\) but with a rotational inertia of \(I\) \[T=2\pi \sqrt { \dfrac { I }{ mgL } } ,\] where \(L\) is the distance from the pivot to the center of mass of the pendulum. Compare the equations of both spring oscillation and simple pendulum, and see how there's an analogy of Hooke's Law in the latter.

Throughout the first half of the \(19^\text{th}\) century, engineers realized that thermal heat was another form of energy, capable of mechanical work. By 1850, the law of conservation of energy was formally stated for the first time, as the first law of thermodynamics . In the last decades of the \(19^\text{th}\) century, Ludwig Boltzmann developed the statistical mechanics theory of heat and entropy, showing the equivalence of kinetic and thermal heat energies, based on the theory that matter was made of atoms. Over time, other forms of energy were recognized such as elastic, electromagnetic, chemical, and nuclear energies, and the law of conservation of energy was generalized to include all such forms of energies.

Firing an Inelastic Shell A tank fires a special hollow shell that has \(N\) neon atoms inside it, so cold (somewhere near absolute zero Kelvin) that relative to the shell the atoms are not moving. The shell has a velocity of \(680\) meters per second, and it slams into a dirt wall and suffers a 100% inelastic collision, where the shell comes to a dead stop. But the neon atoms continue to bounce around inside the shell at the same velocity. What is the temperature of the neon gas inside the shell, after the shell has come to a dead stop? Treat neon as an ideal gas, i.e. point-like particles with mass, and disregard the mass or size of the hollow shell. Neon atoms are \(20.1797\text{ amu},\) or \(\frac { 20.1797 }{ \text{mole} } \) grams, where \(1\text{ mole} = 6.022\times { 10 }^{ 23 }\). The kinetic energy of \(N\) neon atoms travelling at \(680\) meters per second is \[N\frac { 1 }{ 2 } m{ v }^{ 2 }=N\frac { 1 }{ 2 } \left( \frac { 20.1797 }{ 6.022\times { 10 }^{ 23 } } \frac { 1 }{ { 10 }^{ 3 } } \right) { \left( 680 \right) }^{ 2 }\text{ Joules}=N\left( 7.747\times { 10 }^{ -21 } \right)\text{ Joules}.\] Since total energy is conserved , the kinetic energy of the neon atoms has been converted into thermal heat energy. From statistical mechanics of ideal gases, we then have \[N\left( 7.747\times { 10 }^{ -23 } \right)\text{ Joules}=N\frac { 3 }{ 2 } kT \text{ Joules},\] where \(k=1.381\times { 10 }^{ -23 }\text{ Joules/Kelvin}\) is the Boltzmann Constant, and \(T\) is temperature in \(\text{Kelvin}\). From this, we have \[\left( 7.757\times { 10 }^{ -21 } \right) \frac { 2 }{ 3k } =374.088\text{ Kelvin},\] which is just above the boiling point of water. Note that this result does not depend on the number \(N\) of neon atoms so that there could be a gram of neon in the shell, or a single atom of neon. The temperature of an ideal gas depends solely on the mass of the atom and the average velocity of the atoms, is not a form of energy, and does not have the physical dimensions of energy, which thermal heat energy does.

The Rocket Rocket physics describes one kind of rocket that uses fuel as a stored form of chemical energy, which is released in the combustion chamber of the rocket to generate the thrust to propel it. As the fuel is used up, the mass of the rocket drops, while its velocity increases. Using the law of conservation of momentum , velocity of a rocket as a function of mass describes how the following rocket equation can be derived: \[\begin{align} v_f &= v_0 + u\log \frac{M_0}{M_f} , \end{align}\] where \(v_f\) and \(M_f\) are the final velocity and mass, respectively, \(v_0\) and \(M_0\) are the initial velocity and mass, respectively, and \(u\) is the velocity of the rocket exhaust relative to the rocket. \[\text{Indian Polar Satellite Launch Vehicle XL which uses HTPB propellant}\] We can use the law of conservation of energy to derive the same result as follows: Let \(M_0-m\) be the rocket’s mass, where \(m\) is the consumed mass of fuel. Also, let \(v(m)\) be the velocity of the rocket as a function of \(m\), and \(u\) the velocity of the exhaust relative to the rocket. Then we have the following as the total energy of the rocket/exhaust gas system: \[E=\displaystyle \int _{ 0 }^{ m }{ \dfrac { 1 }{ 2 } } \big[ u-v\left( m \right) \big]^2\, dm+\dfrac { 1 }{ 2 } \left( { M }_{ 0 }-m \right) \big[v(m)\big]^2+{ PE }_{ \text{Fuel} }\left( { M }_{ 0 }-m \right), \] where the first term is the kinetic energy of the rocket exhaust gas, the second term is the kinetic energy of the rocket, and the last term \({ PE }_{\text{Fuel} }\left( { M }_{ 0 }-m \right) \) is the potential energy contained in the unburned fuel. The kinetic potential energy of this fuel when it is converted into exhaust gas is simply the kinetic energy it has at velocity \(u\), so we can rewrite this equation as \[E=\displaystyle \int _{ 0 }^{ m }{ \dfrac { 1 }{ 2 } } \big[u-v(m)\big]^2 \, dm+\dfrac { 1 }{ 2 } \left( { M }_{ 0 }-m \right) \big[v(m)\big]^2+\dfrac { 1 }{ 2 } \left( { M }_{ 0 }-m \right) { u }^{ 2 }.\] Since total energy is conserved , we can differentiate both sides by \(m\) with the result \[\begin{align} \dfrac { dE }{ dm } &=0\\\\ \dfrac { 1 }{ 2 }\big[u-v(m)\big]^2-\dfrac { 1 }{ 2 }\big[v(m)\big]^2-\left( {M}_ {0}-m \right) v\left( m \right) \dfrac { dv }{ dm } -\dfrac { 1 }{ 2 } { u }^{ 2 }&=0\\\\ -u-\left( {M}_ {0}-m \right) { \dfrac { dv }{ dm } }&=0\\\\ -\int _{ 0 }^{ m }{ \frac { u }{ {M}_ {0}-m } } \, dm&=\int dv \\\\ u\log\left( \dfrac { {M}_ {0 }}{ {M}_ {0}-m } \right) &={v}_{m}-{v}_{0}, \end{align}\] which agrees with the rocket equation given above. This equation is the most simplified form of rocket physics, which assumes that all the thrust is provided by the exhaust velocity of the combustion gases alone. A real rocket involves other considerations such as combustion gas pressure inside the nozzle.

Mass Energy in Nuclear Reactions A Deuterium atom, \(_1 ^2\ce{H}\), is an isotope of hydrogen that has a nucleus containing one proton and one neutron. Two of them, if collided together at a sufficiently high speed to overcome the Coulomb barrier, can fuse and produce a helium-3 atom \(\left(_2 ^3\ce{He}\right)\) and a free neutron \(\left(_0 ^1\ce{n}\right)\). \(_2 ^3\ce{He}\) is an isotope of helium that has two protons but only one neutron. Excess energy is produced from this fusion, which is carried away as kinetic energy in the products. In atomic mass units \((\text{amu})\), the masses of the nuclei are \[\begin{array}{llll} _1 ^2\ce{H}&=&2.01410179 &\text{ (amu)}\\ _2 ^3\ce{He}&=&3.0160293 &\text{ (amu)}\\ _0 ^1\ce{n}&=&1.008664916 &\text{ (amu)}. \end{array}\] Per Einstein’s \(E=m{ c }^{ 2 }\), mass-to-energy conversion from \(\text{amu}\) to million electron Volts is \[1\text{ amu} = 941.494\text{ MeV}.\] Now, calculate the energy \(E\) in \(\text{MeV}\), produced as the result of the following Deuterium+Deuterium fusion reaction: \[_{ 1 } ^{ 2 }\ce{H} + \ _{ 1 } ^{ 2 }\ce{H} \to \ _{ 2 }^{ 3 }\ce{He}+ \ _0^1\ce{n}+{ E }_{ \text{MeV} }.\] Two deuterium nuclei have a total mass of \(4.02820358\text{ amu},\) but the total mass of \(_2 ^3\ce{He}\) and \(_0 ^1\ce{n}\) is \(4.024694216\text{ amu},\) which is less. The theory of relativity says mass is another form of energy, so since total energy is conserved that missing \(0.00350936\text{ amu}\) mass was converted into energy. Using the mass-to-\(\text{MeV}\) conversion, this works out to about \(E=3.26895\text{ MeV}.\) Note: \(\text{eV}\), or electron volt, is not to be confused with \(\text{V}\), or volt. \(\text{eV}\) has dimensions of energy, or joules, while \(\text{V}\) is joules divided by charge.

If the energy produced is carried away by the products, and if both kinetic energy and momentum are conserved, what is the kinetic energy of the neutron after this fusion reaction? (Disregard the initial kinetic energies of the reactant Deuterium nuclei.) The conservation equations are \[\begin{align} \dfrac { 1 }{ 2 } m_{(^3\ce{He})} v_{(^3\ce{He})}^2+\dfrac { 1 }{ 2 } m_n v_n^2&=E\\ { m }_{ (^3\ce{He}) }{ { v }_{ (^3\ce{He}) } }+{ m }_{ n }{ { v }_{ n } }&=0. \end{align}\] Letting \({ E }_{(^3\ce{He} ) } \) and \({ E }_{ n }\) be the kinetic energies of the products resulting from the fusion, we can restate the two equations from both conservation laws as follows: \[\begin{align} { E }_{ (^3\ce{He}) }+{ E }_{ n }&=E\\ { E }_{(^3\ce{He})}{ m }_{ (^3\ce{He}) }&={ E }_{ n }{ m }_{ n }. \end{align}\] From these, we can work out the kinetic energy of the neutron \[{ E }_{ n }=\dfrac { { m }_{ (^3\ce{He}) } }{ { m }_{ (^3\ce{He}) }+{ m }_{ n } } E,\] which works out to approximately \(2.44969\text{ MeV}\) or \(2.450\text{ MeV}\), which is the usual published value.

ATP synthase, a molecular motor

Spring Pendulum: A Preview of Lagrangian Mechanics Examples given above included a pendulum in a gravitational field, and an oscillating spring-mass system. What if we were to combine them? A massless pendulum arm of relaxed length \(L\) is capable of stretching or compressing lengthwise, and a mass \(m\) is attached to the end of it. Then the position of the mass can be described by two functions to be determined: \[\begin{align} \text{Distance from pivot point } &=L+x(t) \\ \text{Angle from vertical } &=\theta(t). \end{align}\] The total kinetic energy is then the sum of radial and tangential components \[T=\dfrac { 1 }{ 2 } m { \left( \dfrac { dx }{ dt } \right) }^{ 2 }+\dfrac { 1 }{ 2 } m { \left( (L+x)\dfrac { d\theta }{ dt } \right) }^{ 2 } \] and the total potential energy is the sum of gravitational and spring potentials \[V=V\left(x,\theta\right)=-mg(L+x)\cos \theta +\dfrac { 1 }{ 2 } k{ x }^{ 2 },\] where \(g\) is the gravitational acceleration and \(k\) is the spring constant. There are two approaches to classical mechanics (see Lagrangian formulation of mechanics ), which can be briefly summarized as follows: \[\begin{array}{rl} \text{Hamiltonian:} &\mathcal{H} = T+V\\ \text{Lagrangian:} &\mathcal{L}=T-V, \end{array}\] which can be shown to be equivalent through the Legendre transform. In this exercise, we express this spring pendulum by its Lagrangian as follows: \[\mathcal{L}=T-V= \dfrac { 1 }{ 2 } m{ { \dot { x } }^{ 2 }+ \dfrac { 1 }{ 2 } m { \left( (L+x) \dot {\theta } \right) }^{ 2 } }+mg(L+x)\cos \theta -\dfrac { 1 }{ 2 } k{ x }^{ 2 }.\] The two Euler-Lagrange equations for these are then \[\begin{align} \dfrac { d }{ dt } \left( \dfrac { \partial \mathcal {L} }{ \partial \dot { x } } \right) &=\dfrac { \partial \mathcal {L} }{ \partial x } \implies m\ddot { x } =m(L+x){ \dot { \theta } }^{ 2 }+mg\cos \theta –kx\\ \dfrac { d }{ dt } \left( \dfrac { \partial \mathcal {L} }{ \partial \dot { \theta } } \right) &=\dfrac { \partial \mathcal {L} }{ \partial \theta } \implies m(L+x)\ddot { \theta } +2m\dot { x } \dot { \theta } =-mg\sin\theta. \end{align}\] The first is the radial force along the length of the pendulum arm while the second is the tangential force. The complete solution to this pair of differential equations is beyond the scope here, but this example is given to illustrate the generalized utility of treating problems of classical mechanics through the use of kinetic and potential energies, rather than the use of forces and momenta.

The LC Circuit: Via the Lagrangian Approach The classic LC circuit, in the simplest form, consists of an inductor and a capacitor, both of which are capable of storing energy: one electromagnetic and the other electrostatic. This circuit can be analyzed using Kirchhoff’s laws, which is the conventional approach. However, to demonstrate the power of the Lagrangian method, which treats energy as a generalized abstract quantity, the following is a way how the LC circuit can be analyzed in terms of energies alone: Let \(q\left(t\right)\) be the charge as a function of time \(t\). Also, let \(L\) be the inductance of the inductor, and \(C\) the capacitance of the capacitor. Then we can make the analogy of kinetic and potential energies as follows: \[\begin{align} T&=\dfrac { 1 }{ 2 } L{ \left( \dfrac { dq }{ dt } \right) }^{ 2 }\\ V&=\dfrac { 1 }{ 2C } { q }^{ 2 }. \end{align}\] The Lagrangian is then \[\mathcal{L}=\mathcal{L}\left( \dot { q } ,q \right) =T-V=\dfrac { 1 }{ 2 } L{ \dot { q } }^{ 2 }-\dfrac { 1 }{ 2C } { q }^{ 2 }.\] The Euler-Lagrange equation is then \[\dfrac { d }{ dt } \left( \dfrac { \mathcal{L} }{ \partial \dot { q } } \right) -\dfrac { \mathcal{L} }{ \partial q } =0\implies \ddot { q } +\dfrac { q }{ LC } =0,\] which yields the same solution as can be found by using Kirchhoff’s laws \[q\left( t \right) ={ q }_{ 0 }\cos\left( \omega t \right) \] given that \(q\left(0\right)=0, \dot{q}\left(0\right)=0\) and \(\omega =\frac { 1 }{ \sqrt { LC } } .\)

The total mechanical (kinetic + potential) energy of a free falling object are always equal to the potential energy at the height from which it was dropped from rest. Is this statement true or false?

Neglect air resistance.

An object is dropped from rest from a height of \(h\). What is the velocity of the object in the middle of its journey, i.e. when the object is at half its original distance to its destination?

Two bodies \(A\) and \(B\) have an equal momentum. But the mass of \(A\) is less than the mass of \(B\).

Which of the two will have more kinetic energy?

This problem is posted in connection to the upcoming collaboration party hosted by me! Interested people may come forward (just ping me on slack).

Problem Loading...

Note Loading...

Set Loading...

JEE-IIT-NCERT Physics & Math

Widget atas posting, conservation of mechanical energy problems and solutions, post a comment for "conservation of mechanical energy problems and solutions".

See Our New JEE Book on Amazon

Conservation of Mechanical Energy

Mechanical energy of a system is the sum of its kinetic and potential energies. In the absence of non-conservative forces, the total mechanical energy of a system remains constant. The conservation of mechanical energy is a useful tool for solving problems.

Problems from IIT JEE

Problem (JEE Mains 2006): The potential energy of a 1 kg particle free to move along the $x$-axis is given by \begin{align} U(x)=\left(\frac{x^4}{4}-\frac{x^2}{2}\right)\mathrm{J}.\nonumber \end{align} The total mechanical energy of the particle 2 J. Then, the maximum speed (in m/s) is_______?

Solution: The variation of potential energy $U(x)={x^4}/{4}-{x^2}/{2}$ with $x$ is shown in the figure. It has a local maxima at $x=0$ and two global minima at $x=-1$ and $x=1$. You can get these points by solving $\mathrm{d}U/\mathrm{d}x=0$. The minimum value of potential energy is, \begin{align} U_\text{min} & =U(x\!=\!-1) \\ &=U(x\!=\!1) \\ &=-1/4\;\mathrm{J}.\nonumber \end{align}

The total energy of the particle is $E=2$ J. The kinetic energy, $K=E-U$, is maximum when the potential energy is minimum. The maximum kinetic energy of the particle is, \begin{align} K_\text{max} & =E-U_\text{min} \\ &=2-(-1/4)=9/4\;\mathrm{J}.\nonumber \end{align} The maximum velocity of the particle is, \begin{align} v_\text{max} & =\sqrt{2K_\text{max}/m} \\ &=\sqrt{2(9/4)/(1)} \\ &=3/\sqrt{2}\;\mathrm{J}.\nonumber \end{align}

This problem has beautiful hidden concepts. Let us explore some of them. Look at the figure. What is the particle's kinetic energy at $x=-2$ and $x=2$? It is zero. The particle comes to a momentary halt at these points and returns. It is traveling in a closed path between $x=-2$ and $x=2$. The particle is prohibited from entering the region $x 2$. The kinetic energy in these regions is negative, which is meaningless.

Let a particle of total energy $E=-1/2$ J is released near $x=1$. The particle will travel in a closed path between $x_\text{min}$ and $x_\text{max}$. Can you find the values of $x_\text{min}$ and $x_\text{max}$?

• $y={-5}\;\mathrm{cm}$
• $y={20}\;\mathrm{cm}$
• $y={5}\;\mathrm{cm}$
• $y={-20}\;\mathrm{cm}$

Solution: There is no external force on the system in the $x\text{-}y$ plane. Hence, linear momentum of the system is conserved along $x$ and $y$ directions, separately. Initially, the particle was moving along $x$ axis with a speed $v_x$. Hence, coordinates of its centre of mass varies with time $t$ as, \begin{align} x_{c}=v_x t, \qquad y_{c}=0.\nonumber \end{align} By conservation of linear momentum, $y_{c}$ remains constant (here zero) after the collision i.e., \begin{align} y_{c}=\frac{({m}/{4}) 15+({3m}/{4})y}{{m}/{4}+{3m}/{4}}=0. \end{align} Solve to get $y={-5}\;\mathrm{cm}$.

• Kinetic and Potential Energy
• Work-Energy Theorem
• The potential energy of spring
• Conservative & Nonconservative Forces
• Elastic and Inelastic Collisions

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

8.8: Sample problems and solutions

• Last updated
• Save as PDF
• Page ID 19419

Exercise \(\PageIndex{1}\)

A ball of mass \(m\) is dropped onto a vertical spring with spring constant \(k\) . The spring will compress until the ball comes to rest. How much will it compress if the ball is dropped from a height \(h\) above the spring?

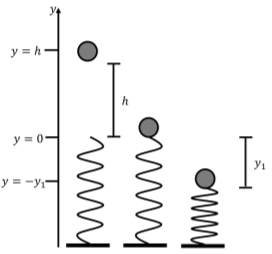
The two forces acting on the ball are gravity and the spring force. Both are conservative, so we can use conservation of mechanical energy. We will find the energy of the ball when it is at a height \(h\) above the spring, and the energy of the ball when the spring is fully compressed. Then, we will use conservation of mechanical energy to determine the compression of the spring.

Remember that the total mechanical energy is the sum of the total potential energy and the kinetic energy, \(E=U+K\) . Let’s call the initial position of the ball \(A\) and the final position of the ball \(B\) . You will notice that we set up our coordinate system so that \(y\) is positive upwards, with \(y=0\) at the point where the ball comes into contact with the spring. We choose to define both the gravitational potential energy and spring potential energy so that they are zero at \(y=0\) .

Since the ball starts from rest, its kinetic energy is zero at position \(A\) . At this point, the ball is not touching the spring, so the potential energy from the spring force is zero. The mechanical energy of the ball at position \(A\) is simply equal to its gravitational potential energy: \[\begin{aligned} E_A&=U_A+K_A\\ E_A&=mgh\end{aligned}\] At position \(B\) , the ball is again at rest, so the kinetic energy of the ball is zero. Now that the ball is in contact with the spring, it will experience a force from the spring that can be modeled with a potential energy \(U(y)=\frac{1}{2}ky_1^2\) , where \(y_1\) is the distance between the rest position of the spring and its compressed length. At point \(B\) ( \(y=-y_1\) ), the ball will have both spring and gravitational potential energy, so its mechanical energy at position \(B\) is given by: \[\begin{aligned} E_B&=U_B+K_B=U_B\\ U_B&=mg(-y_1)+\frac{1}{2}ky_1^2\\ E_B&=-mgy_1+\frac{1}{2}ky_1^2\end{aligned}\] Since mechanical energy is conserved in this system (no non-conservative forces are doing work), we can now set \(E_A=E_B\) and solve for \(y_1\) : \[\begin{aligned} E_A&=E_B\\ mgh&=-mgy_1+\frac{1}{2}ky_1^2\\ 0&=\frac{1}{2}ky_1^2-mgy_1-mgh\\\end{aligned}\] where in the last line we rewrote the expression as a quadratic equation. We can solve for \(y_1\) with the quadratic formula: \[\begin{aligned} y_1=\frac{mg\pm\sqrt{(mg)^2-4(1/2k)(-mgh)}}{k}\\ y_1=\frac{mg\pm\sqrt{mg(mg+2kh)}}{k}\end{aligned}\] We now have an expression for the amount the spring is compressed, \(y_1\) , in terms of our known values.

Exercise \(\PageIndex{2}\)

A simple pendulum consists of a mass \(m\) connected to a string of length \(L\) . The pendulum is released from an angle \(\theta_0\) from the vertical. Use conservation of energy to find an expression for the velocity of the mass as a function of the angle.

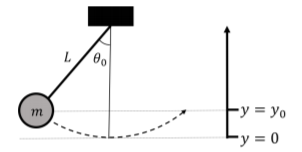
We are going to find a general expression for the energy of the system, and then use this expression to find the velocity at any point. There are two forces acting on the mass:

The force of tension (from the string). This force is perpendicular to the direction of motion at any point, so it does no work on the mass.

The force of gravity, which has a potential energy function given by \(U(y)=mgy\) . We choose the gravitational potential energy to be zero when the pendulum hangs vertically (when \(\theta=0\) and \(y=0\) ).

The mechanical energy of the mass is conserved, and at any point is given by the sum of its kinetic and its gravitational potential energies: \[\begin{aligned} E=mgy+\frac{1}{2}mv^2\end{aligned}\] We want to find the velocity as a function of \(\theta\) , so we need to write \(y\) in terms of \(\theta\) . As you may recall from Problem 7.6.2 , we saw that from the geometry of the problem, we can express the height of the mass as \(y=L-L\cos\theta\) , or \(L(1-\cos\theta)\) , where \(y\) is the height as measured from the bottom point of the motion. You can refer to Figure 7.6.4 to refresh your memory. The energy at any point is then: \[\begin{aligned} E=mgL(1-\cos\theta)+\frac{1}{2}mv^2\end{aligned}\] Conservation of energy tells us that the total energy at any point must be the same as the initial energy. So, we can use our initial conditions to find the total energy of the system. The mass starts from rest (initial kinetic energy is zero) an angle \(\theta_0\) above the vertical: \[\begin{aligned} E&=mgL(1-\cos\theta)+\frac{1}{2}mv^2\\ E_{initial}&=mgL(1-\cos\theta_0)\end{aligned}\] Now that we have found the total energy of the system, we can write our general expression for the energy of the system at any point: \[\begin{aligned} E&=mgL(1-\cos\theta)+\frac{1}{2}mv^2\\ mgL(1-\cos\theta_0)&=mgL(1-\cos\theta)+\frac{1}{2}mv^2\end{aligned}\] All that’s left to do is simplify the expression and rearrange for \(v\) : \[\begin{aligned} mgL(1-\cos\theta_0)&=mgL(1-\cos\theta)+\frac{1}{2}mv^2\\ gL(1-\cos\theta_0)-gL(1-\cos\theta)&=\frac{1}{2}v^2\\ gL-gL\cos\theta_0-gL+gL\cos\theta&=\frac{1}{2}v^2\\ gL(\cos\theta-\cos\theta_0)&=\frac{1}{2}v^2\\ \therefore v&=\sqrt{2gl(\cos\theta-\cos\theta_0)}\end{aligned}\]

Discussion:

We can see from this expression that the speed will be maximized when \(\cos\theta\) is maximized, which will occur when \(\theta=0\) (when the pendulum is vertical). This is as we expected. We can also see that we will get an imaginary number if the magnitude of \(\theta\) is greater than \(\theta_0\) , showing that the motion is constrained between \(-\theta_0\) and \(\theta_0\) . Finally, we showed that the velocity of the pendulum does not depend on the mass!

Exercise \(\PageIndex{3}\)

A block of mass \(m\) sits on a frictionless horizontal surface. It is attached to a wall by a spring with a spring constant \(k\) . The mass is pushed so as to compress the spring and then it is released (Figure \(\PageIndex{3}\)). Use the Lagrangian formalism to find an equation of motion for the mass/spring system (i.e. use the Lagrangian to determine the acceleration of the mass).

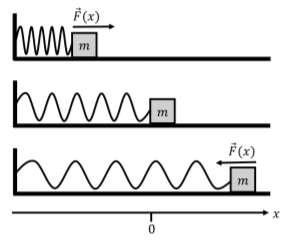
We are going to find an equation of motion of the system using the Lagrangian method. We choose to use a one dimension coordinate system, with the \(x\) axis defined to be co-linear with the spring, positive in the direction where the spring is extended, and set the origin to be located at the rest position of the spring. The kinetic energy and potential energy of the mass are given by \[\begin{aligned} K&=\frac{1}{2}mv_x^2\\ U&=\frac{1}{2}kx^2\end{aligned}\] since the only force exerted on the mass that can do work is the force from the spring. We have chosen the potential energy to be zero at \(x=0\) . The Lagrangian for this system is: \[\begin{aligned} L&=K-U\\ L&=\frac{1}{2}mv_x^2-\frac{1}{2}kx^2\end{aligned}\]

The Euler-Lagrange equation in one dimension is:

\[\begin{aligned} \frac{d}{dt}\left(\frac{\partial L}{\partial v_{x}}\right)-\frac{\partial L}{\partial x} = 0\end{aligned}\]

We can calculate the terms of the Euler-Lagrange equation:

\[\begin{aligned} \frac{\partial L}{\partial v_{x}}&=\frac{\partial}{\partial v_{x}}\left(\frac{1}{2}mv_x^2-\frac{1}{2}kx^2\right)\\ &=mv_x\\ \therefore \frac{d}{dt}\left(\frac{\partial L}{\partial v_{x}}\right)&=\frac{d}{dt}(mv_x)\\ &=ma_x\\ \textrm{and}\qquad \frac{\partial L}{\partial x}&=\left(\frac{1}{2}mv_x^2-\frac{1}{2}kx^2\right)\\ &=-kx\end{aligned}\]

and then put them together to get:

\[\begin{aligned} \frac{d}{dt}\left(\frac{\partial L}{\partial v_{x}}\right)-\frac{\partial L}{\partial x} &= 0\\ \therefore ma_x&=-kx\\\end{aligned}\]

We can see that this equation of motion is equivalent to Newton’s Second Law.

Large-scale blackouts occur in Rostov-on-Don, Russia, power supply schedule introduced

Electricity disappeared in several areas of Russian Rostov-on-Don on the evening of 13 January.

Source: local publication 161.ru

Details: With reference to readers and social media, the publication reports that residents of the Zapadny, Red Aksai, Nakhichevan, Aleksandrovka, Chkalovsky [microdistricts and residential areas in the city of Rostov-on-Don] and the centre of Rostov were left without power. Also, electricity disappeared in parts of Belaya Kalitva and Bataysk.

The power was turned off at about 21:30. According to the management company Komservice, the streets of Magnitogorsk, Zavodskaya, Zvivistaya and part of Zapadnaya were de-energized. In their Telegram channel, the companies specify that the shutdown would last 2-3 hours.

"Rosseti [Russian Networks – ed.] introduced a schedule of temporary restriction of electricity supply, which is why in particular our consumers were left without power (our substations are powered by Rosseti equipment)," Donenergo commented on the situation.

Sergey Sizikov, Minister of Housing and Public Utilities of Rostov Oblast, reported in his Telegram channel that, according to power engineers, electricity is going to be restored completely by 23:30.

Quote: " Due to technological violations in high-voltage networks, the Regional Dispatch Directorate for Rostov Oblast of the RAO UES [electric power holding company in Russia – ed.] of Russia decided to introduce temporary shutdown schedules in the amount of 270 MW in a number of districts of Rostov Oblast. They plan to restore the normal power supply scheme in the near future," Rosseti Yug (Russian Networks South) commented on the shutdown.

Updated: At 23:58 Moscow time (22:58 Kyiv time), Rosseti South said that electricity was returned to the houses at 23:37.

Support UP or become our patron !

• TPC and eLearning
• Read Watch Interact
• What's NEW at TPC?
• Practice Review Test
• Teacher-Tools
• Subscription Selection
• Seat Calculator
• Ad Free Account
• Edit Profile Settings
• Classes (Version 2)
• Student Progress Edit
• Task Properties
• Export Student Progress
• Task, Activities, and Scores
• Metric Conversions Questions
• Metric System Questions
• Metric Estimation Questions
• Significant Digits Questions
• Proportional Reasoning
• Acceleration
• Distance-Displacement
• Dots and Graphs
• Graph That Motion
• Match That Graph
• Name That Motion
• Motion Diagrams
• Pos'n Time Graphs Numerical
• Pos'n Time Graphs Conceptual
• Up And Down - Questions
• Balanced vs. Unbalanced Forces
• Change of State
• Force and Motion
• Mass and Weight
• Match That Free-Body Diagram
• Net Force (and Acceleration) Ranking Tasks
• Newton's Second Law
• Normal Force Card Sort
• Recognizing Forces
• Air Resistance and Skydiving
• Solve It! with Newton's Second Law
• Which One Doesn't Belong?
• Component Addition Questions
• Head-to-Tail Vector Addition
• Projectile Mathematics
• Trajectory - Angle Launched Projectiles
• Trajectory - Horizontally Launched Projectiles
• Vector Addition
• Vector Direction
• Which One Doesn't Belong? Projectile Motion
• Forces in 2-Dimensions
• Being Impulsive About Momentum
• Explosions - Law Breakers
• Hit and Stick Collisions - Law Breakers
• Case Studies: Impulse and Force
• Impulse-Momentum Change Table
• Keeping Track of Momentum - Hit and Stick
• Keeping Track of Momentum - Hit and Bounce
• What's Up (and Down) with KE and PE?
• Energy Conservation Questions
• Energy Dissipation Questions
• Energy Ranking Tasks
• LOL Charts (a.k.a., Energy Bar Charts)
• Match That Bar Chart
• Words and Charts Questions
• Name That Energy
• Stepping Up with PE and KE Questions
• Case Studies - Circular Motion
• Circular Logic
• Forces and Free-Body Diagrams in Circular Motion
• Gravitational Field Strength
• Universal Gravitation
• Angular Position and Displacement
• Linear and Angular Velocity
• Angular Acceleration
• Rotational Inertia
• Balanced vs. Unbalanced Torques
• Getting a Handle on Torque
• Torque-ing About Rotation
• Properties of Matter
• Fluid Pressure
• Buoyant Force
• Sinking, Floating, and Hanging
• Pascal's Principle
• Flow Velocity
• Bernoulli's Principle
• Balloon Interactions
• Charge and Charging
• Charge Interactions
• Charging by Induction
• Conductors and Insulators
• Coulombs Law
• Electric Field
• Electric Field Intensity
• Polarization
• Case Studies: Electric Power
• Know Your Potential
• Light Bulb Anatomy
• I = ∆V/R Equations as a Guide to Thinking
• Parallel Circuits - ∆V = I•R Calculations
• Resistance Ranking Tasks
• Series Circuits - ∆V = I•R Calculations
• Series vs. Parallel Circuits
• Equivalent Resistance
• Period and Frequency of a Pendulum
• Pendulum Motion: Velocity and Force
• Energy of a Pendulum
• Period and Frequency of a Mass on a Spring
• Horizontal Springs: Velocity and Force
• Vertical Springs: Velocity and Force
• Energy of a Mass on a Spring
• Decibel Scale
• Frequency and Period
• Closed-End Air Columns
• Name That Harmonic: Strings
• Rocking the Boat
• Wave Basics
• Matching Pairs: Wave Characteristics
• Wave Interference
• Waves - Case Studies
• Color Addition and Subtraction
• Color Filters
• If This, Then That: Color Subtraction
• Light Intensity
• Color Pigments
• Converging Lenses
• Curved Mirror Images
• Law of Reflection
• Refraction and Lenses
• Total Internal Reflection
• Who Can See Who?
• Formulas and Atom Counting
• Atomic Models
• Bond Polarity
• Entropy Questions
• Cell Voltage Questions
• Heat of Formation Questions
• Reduction Potential Questions
• Oxidation States Questions
• Measuring the Quantity of Heat
• Hess's Law
• Oxidation-Reduction Questions
• Galvanic Cells Questions
• Thermal Stoichiometry
• Molecular Polarity
• Quantum Mechanics
• Balancing Chemical Equations
• Bronsted-Lowry Model of Acids and Bases
• Classification of Matter
• Collision Model of Reaction Rates
• Density Ranking Tasks
• Dissociation Reactions
• Complete Electron Configurations
• Elemental Measures
• Enthalpy Change Questions
• Equilibrium Concept
• Equilibrium Constant Expression
• Equilibrium Calculations - Questions
• Equilibrium ICE Table
• Ionic Bonding
• Lewis Electron Dot Structures
• Limiting Reactants
• Line Spectra Questions
• Measurement and Numbers
• Metals, Nonmetals, and Metalloids
• Metric Estimations
• Metric System
• Molarity Ranking Tasks
• Mole Conversions
• Name That Element
• Names to Formulas
• Names to Formulas 2
• Nuclear Decay
• Particles, Words, and Formulas
• Periodic Trends
• Precipitation Reactions and Net Ionic Equations
• Pressure Concepts
• Pressure-Temperature Gas Law
• Pressure-Volume Gas Law
• Chemical Reaction Types
• Significant Digits and Measurement
• States Of Matter Exercise
• Stoichiometry - Math Relationships
• Subatomic Particles
• Spontaneity and Driving Forces
• Gibbs Free Energy
• Volume-Temperature Gas Law
• Acid-Base Properties
• Energy and Chemical Reactions
• Chemical and Physical Properties
• Valence Shell Electron Pair Repulsion Theory
• Writing Balanced Chemical Equations
• Mission CG1
• Mission CG10
• Mission CG2
• Mission CG3
• Mission CG4
• Mission CG5
• Mission CG6
• Mission CG7
• Mission CG8
• Mission CG9
• Mission EC1
• Mission EC10
• Mission EC11
• Mission EC12
• Mission EC2
• Mission EC3
• Mission EC4
• Mission EC5
• Mission EC6
• Mission EC7
• Mission EC8
• Mission EC9
• Mission RL1
• Mission RL2
• Mission RL3
• Mission RL4
• Mission RL5
• Mission RL6
• Mission KG7
• Mission RL8
• Mission KG9
• Mission RL10
• Mission RL11
• Mission RM1
• Mission RM2
• Mission RM3
• Mission RM4
• Mission RM5
• Mission RM6
• Mission RM8
• Mission RM10
• Mission LC1
• Mission RM11
• Mission LC2
• Mission LC3
• Mission LC4
• Mission LC5
• Mission LC6
• Mission LC8
• Mission SM1
• Mission SM2
• Mission SM3
• Mission SM4
• Mission SM5
• Mission SM6
• Mission SM8
• Mission SM10
• Mission KG10
• Mission SM11
• Mission KG2
• Mission KG3
• Mission KG4
• Mission KG5
• Mission KG6
• Mission KG8
• Mission KG11
• Mission F2D1
• Mission F2D2
• Mission F2D3
• Mission F2D4
• Mission F2D5
• Mission F2D6
• Mission KC1
• Mission KC2
• Mission KC3
• Mission KC4
• Mission KC5
• Mission KC6
• Mission KC7
• Mission KC8
• Mission AAA
• Mission SM9
• Mission LC7
• Mission LC9
• Mission NL1
• Mission NL2
• Mission NL3
• Mission NL4
• Mission NL5
• Mission NL6
• Mission NL7
• Mission NL8
• Mission NL9
• Mission NL10
• Mission NL11
• Mission NL12
• Mission MC1
• Mission MC10
• Mission MC2
• Mission MC3
• Mission MC4
• Mission MC5
• Mission MC6
• Mission MC7
• Mission MC8
• Mission MC9
• Mission RM7
• Mission RM9
• Mission RL7
• Mission RL9
• Mission SM7
• Mission SE1
• Mission SE10
• Mission SE11
• Mission SE12
• Mission SE2
• Mission SE3
• Mission SE4
• Mission SE5
• Mission SE6
• Mission SE7
• Mission SE8
• Mission SE9
• Mission VP1
• Mission VP10
• Mission VP2
• Mission VP3
• Mission VP4
• Mission VP5
• Mission VP6
• Mission VP7
• Mission VP8
• Mission VP9
• Mission WM1
• Mission WM2
• Mission WM3
• Mission WM4
• Mission WM5
• Mission WM6
• Mission WM7
• Mission WM8
• Mission WE1
• Mission WE10
• Mission WE2
• Mission WE3
• Mission WE4
• Mission WE5
• Mission WE6
• Mission WE7
• Mission WE8
• Mission WE9
• Vector Walk Interactive
• Name That Motion Interactive
• Kinematic Graphing 1 Concept Checker
• Kinematic Graphing 2 Concept Checker
• Graph That Motion Interactive
• Two Stage Rocket Interactive
• Rocket Sled Concept Checker
• Force Concept Checker
• Free-Body Diagrams Concept Checker
• Free-Body Diagrams The Sequel Concept Checker
• Skydiving Concept Checker
• Elevator Ride Concept Checker
• Vector Addition Concept Checker
• Vector Walk in Two Dimensions Interactive
• Name That Vector Interactive
• River Boat Simulator Concept Checker
• Projectile Simulator 2 Concept Checker
• Projectile Simulator 3 Concept Checker
• Hit the Target Interactive
• Turd the Target 1 Interactive
• Turd the Target 2 Interactive
• Balance It Interactive
• Go For The Gold Interactive
• Egg Drop Concept Checker
• Fish Catch Concept Checker
• Exploding Carts Concept Checker
• Collision Carts - Inelastic Collisions Concept Checker
• Its All Uphill Concept Checker
• Stopping Distance Concept Checker
• Chart That Motion Interactive
• Roller Coaster Model Concept Checker
• Uniform Circular Motion Concept Checker
• Horizontal Circle Simulation Concept Checker
• Vertical Circle Simulation Concept Checker
• Race Track Concept Checker
• Gravitational Fields Concept Checker
• Orbital Motion Concept Checker
• Angular Acceleration Concept Checker
• Balance Beam Concept Checker
• Torque Balancer Concept Checker
• Aluminum Can Polarization Concept Checker
• Charging Concept Checker
• Name That Charge Simulation
• Coulomb's Law Concept Checker
• Electric Field Lines Concept Checker
• Put the Charge in the Goal Concept Checker
• Circuit Builder Concept Checker (Series Circuits)
• Circuit Builder Concept Checker (Parallel Circuits)
• Circuit Builder Concept Checker (∆V-I-R)
• Circuit Builder Concept Checker (Voltage Drop)
• Equivalent Resistance Interactive
• Pendulum Motion Simulation Concept Checker
• Mass on a Spring Simulation Concept Checker
• Particle Wave Simulation Concept Checker
• Boundary Behavior Simulation Concept Checker
• Slinky Wave Simulator Concept Checker
• Simple Wave Simulator Concept Checker
• Wave Addition Simulation Concept Checker
• Standing Wave Maker Simulation Concept Checker
• Color Addition Concept Checker
• Painting With CMY Concept Checker
• Stage Lighting Concept Checker
• Filtering Away Concept Checker
• InterferencePatterns Concept Checker
• Young's Experiment Interactive
• Plane Mirror Images Interactive
• Who Can See Who Concept Checker
• Optics Bench (Mirrors) Concept Checker
• Name That Image (Mirrors) Interactive
• Refraction Concept Checker
• Total Internal Reflection Concept Checker
• Optics Bench (Lenses) Concept Checker
• Kinematics Preview
• Velocity Time Graphs Preview
• Moving Cart on an Inclined Plane Preview
• Stopping Distance Preview
• Cart, Bricks, and Bands Preview
• Fan Cart Study Preview
• Friction Preview
• Coffee Filter Lab Preview
• Friction, Speed, and Stopping Distance Preview
• Up and Down Preview
• Projectile Range Preview
• Ballistics Preview
• Juggling Preview
• Marshmallow Launcher Preview
• Air Bag Safety Preview
• Colliding Carts Preview
• Collisions Preview
• Engineering Safer Helmets Preview
• Push the Plow Preview
• Its All Uphill Preview
• Energy on an Incline Preview
• Modeling Roller Coasters Preview
• Hot Wheels Stopping Distance Preview
• Ball Bat Collision Preview
• Energy in Fields Preview
• Weightlessness Training Preview
• Roller Coaster Loops Preview
• Universal Gravitation Preview
• Keplers Laws Preview
• Kepler's Third Law Preview
• Charge Interactions Preview
• Sticky Tape Experiments Preview
• Wire Gauge Preview
• Voltage, Current, and Resistance Preview
• Light Bulb Resistance Preview
• Series and Parallel Circuits Preview
• Thermal Equilibrium Preview
• Linear Expansion Preview
• Heating Curves Preview
• Electricity and Magnetism - Part 1 Preview
• Electricity and Magnetism - Part 2 Preview
• Vibrating Mass on a Spring Preview
• Period of a Pendulum Preview
• Wave Speed Preview
• Slinky-Experiments Preview
• Standing Waves in a Rope Preview
• Sound as a Pressure Wave Preview
• DeciBel Scale Preview
• DeciBels, Phons, and Sones Preview
• Sound of Music Preview
• Shedding Light on Light Bulbs Preview
• Models of Light Preview
• Electromagnetic Radiation Preview
• Electromagnetic Spectrum Preview
• EM Wave Communication Preview
• Digitized Data Preview
• Light Intensity Preview
• Concave Mirrors Preview
• Object Image Relations Preview
• Snells Law Preview
• Reflection vs. Transmission Preview
• Magnification Lab Preview
• Reactivity Preview
• Ions and the Periodic Table Preview
• Periodic Trends Preview
• Reaction Rates Preview
• Ammonia Factory Preview
• Stoichiometry Preview
• Gaining Teacher Access
• Tasks and Classes
• Tasks - Classic
• Subscription
• Subscription Locator
• 1-D Kinematics
• Newton's Laws
• Vectors - Motion and Forces in Two Dimensions
• Momentum and Its Conservation
• Work and Energy
• Circular Motion and Satellite Motion
• Thermal Physics
• Static Electricity
• Electric Circuits
• Vibrations and Waves
• Sound Waves and Music
• Light and Color
• Reflection and Mirrors
• About the Physics Interactives
• Task Tracker
• Usage Policy
• Newtons Laws
• Vectors and Projectiles
• Forces in 2D
• Momentum and Collisions
• Circular and Satellite Motion
• Balance and Rotation
• Electromagnetism
• Waves and Sound
• Forces in Two Dimensions
• Work, Energy, and Power
• Circular Motion and Gravitation
• Sound Waves
• 1-Dimensional Kinematics
• Circular, Satellite, and Rotational Motion
• Einstein's Theory of Special Relativity
• Waves, Sound and Light
• QuickTime Movies
• About the Concept Builders
• Pricing For Schools
• Directions for Version 2
• Measurement and Units
• Relationships and Graphs
• Rotation and Balance
• Vibrational Motion
• Reflection and Refraction
• Teacher Accounts
• Task Tracker Directions
• Kinematic Concepts
• Kinematic Graphing
• Wave Motion
• Sound and Music
• About CalcPad
• 1D Kinematics
• Vectors and Forces in 2D
• Simple Harmonic Motion
• Rotational Kinematics
• Rotation and Torque
• Rotational Dynamics
• Electric Fields, Potential, and Capacitance
• Transient RC Circuits
• Light Waves
• Units and Measurement
• Stoichiometry
• Molarity and Solutions
• Thermal Chemistry
• Acids and Bases
• Kinetics and Equilibrium
• Solution Equilibria
• Oxidation-Reduction
• Nuclear Chemistry
• NGSS Alignments
• 1D-Kinematics
• Projectiles
• Circular Motion
• Magnetism and Electromagnetism
• Graphing Practice
• About the ACT
• ACT Preparation
• For Teachers
• Other Resources
• Newton's Laws of Motion
• Work and Energy Packet
• Static Electricity Review
• Solutions Guide
• Solutions Guide Digital Download
• Motion in One Dimension
• Work, Energy and Power
• Frequently Asked Questions
• Purchasing the Download
• Purchasing the CD
• Purchasing the Digital Download
• About the NGSS Corner
• NGSS Search
• Force and Motion DCIs - High School
• Energy DCIs - High School
• Wave Applications DCIs - High School
• Force and Motion PEs - High School
• Energy PEs - High School
• Wave Applications PEs - High School
• Crosscutting Concepts
• The Practices
• Physics Topics
• NGSS Corner: Activity List
• NGSS Corner: Infographics
• About the Toolkits
• Position-Velocity-Acceleration
• Position-Time Graphs
• Velocity-Time Graphs
• Newton's First Law
• Newton's Second Law
• Newton's Third Law
• Terminal Velocity
• Projectile Motion
• Forces in 2 Dimensions
• Impulse and Momentum Change
• Momentum Conservation
• Work-Energy Fundamentals
• Work-Energy Relationship
• Roller Coaster Physics
• Satellite Motion
• Electric Fields
• Circuit Concepts
• Series Circuits
• Parallel Circuits
• Describing-Waves
• Wave Behavior Toolkit
• Standing Wave Patterns
• Resonating Air Columns
• Wave Model of Light
• Plane Mirrors
• Curved Mirrors
• Teacher Guide
• Using Lab Notebooks
• Current Electricity
• Light Waves and Color
• Reflection and Ray Model of Light
• Refraction and Ray Model of Light
• Classes (Legacy Version)
• Teacher Resources
• Subscriptions

• Newton's Laws
• Einstein's Theory of Special Relativity
• About Concept Checkers
• School Pricing
• Newton's Laws of Motion
• Newton's First Law
• Newton's Third Law
• Analysis of Situations Involving External Forces
• Internal vs. External Forces
• Analysis of Situations in Which Mechanical Energy is Conserved
• Application and Practice Questions
• Bar Chart Illustrations

Relating Work to Energy

The quantitative relationship between work and mechanical energy is expressed by the following equation:

The equation states that the initial amount of total mechanical energy (TME i ) plus the work done by external forces (W ext ) is equal to the final amount of total mechanical energy (TME f ). A few notes should be made about the above equation. First, the mechanical energy can be either potential energy (in which case it could be due to springs or gravity ) or kinetic energy . Given this fact, the above equation can be rewritten as

The second note that should be made about the above equation is that the work done by external forces can be a positive or a negative work term . Whether the work term takes on a positive or a negative value is dependent upon the angle between the force and the motion. Recall from Lesson 1 that the work is dependent upon the angle between the force and the displacement vectors. If the angle is 180 degrees as it occasionally is, then the work term will be negative. If the angle is 0 degrees, then the work term will be positive.

Raising a Barbell Vertically

To begin our investigation of the work-energy relationship, we will investigate situations involving work being done by external forces (nonconservative forces). Consider a weightlifter who applies an upwards force (say 1000 N) to a barbell to displace it upwards a given distance (say 0.25 meters) at a constant speed. The initial energy plus the work done by the external force equals the final energy. If the barbell begins with 1500 Joules of energy (this is just a made up value) and the weightlifter does 250 Joules of work ( F•d•cosine of angle = 1000 N•0.25 m•cosine 0 degrees = 250 J), then the barbell will finish with 1750 Joules of mechanical energy. The final amount of mechanical energy (1750 J) is equal to the initial amount of mechanical energy (1500 J) plus the work done by external forces (250 J).

Catching a Baseball

Now consider a baseball catcher who applies a rightward force (say 6000 N) to a leftward moving baseball to bring it from a high speed to a rest position over a given distance (say 0.10 meters). The initial energy plus the work done by the external force equals the final energy. If the ball begins with 605 Joules of energy (this is just another made up value), and the catcher does -600 Joules of work ( F•d•cosine of angle = 6000 N•0.10 m•cosine 180 degrees = -600 J), then the ball will finish with 5 Joules of mechanical energy. The final energy (5 J) is equal to the initial energy (605 J) plus the work done by external forces (-600 J).

A Skidding Car

Now consider a car that is skidding from a high speed to a lower speed. The force of friction between the tires and the road exerts a leftward force (say 8000 N) on the rightward moving car over a given distance (say 30 m). The initial energy plus the work done by the external force equals the final energy. If the car begins with 320 000 Joules of energy (this is just another made up value), and the friction force does -240 000 Joules of work ( F•d•cosine of angle = 8000 N•30 m•cosine 180 degrees = -240 000 J), then the car will finish with 80 000 Joules of mechanical energy. The final energy (80 000 J) is equal to the initial energy (320 000 J) plus the work done by external forces (-240 000 J).

Pulling a Cart Up an Incline at Constant Speed

As a final example, consider a cart being pulled up an inclined plane at constant speed by a student during a Physics lab. The applied force on the cart (say 18 N) is directed parallel to the incline to cause the cart to be displaced parallel to the incline for a given displacement (say 0.7 m). The initial energy plus the work done by the external force equals the final energy. If the cart begins with 0 Joules of energy (this is just another made up value), and the student does 12.6 Joules of work ( F•d•cosine of angle = 18 N•0.7 m•cosine 0 degrees = 12.6 J), then the cart will finish with 12.6 Joules of mechanical energy. The final energy (12.6 J) is equal to the initial energy (0 J) plus the work done by external forces (12.6 J).

In each of these examples, an external force does work upon an object over a given distance to change the total mechanical energy of the object. If the external force (or nonconservative force) does positive work , then the object gains mechanical energy. The amount of energy gained is equal to the work done on the object. If the external force (or nonconservative force) does negative work , then the object loses mechanical energy. The amount of mechanical energy lost is equal to the work done on the object. In general, the total mechanical energy of the object in the initial state (prior to the work being done) plus the work done equals the total mechanical energy in the final state.

Your Turn to Try It

The work-energy relationship presented here can be combined with the expressions for potential and kinetic energy to solve complex problems. Like all complex problems, they can be made simple if first analyzed from a conceptual viewpoint and broken down into parts. In other words, avoid treating work-energy problems as mere mathematical problems. Rather, engage your mind and utilize your understanding of physics concepts to approach the problem. Ask "What forms of energy are present initially and finally?" and "Based on the equations, how much of each form of energy is present initially and finally?" and "Is work being done by external forces?" Use this approach on the following three practice problems. After solving, click the button to view the answers.

We Would Like to Suggest ...

PE = 0 J (the car's height is zero) KE = 0.5*1000*(25)^2 = 312 500 J

PE = 0 J (the car's height is zero) KE = 0 J (the car's speed is zero)

The work done is (8000 N) • (d) • cos 180 = - 8000*d

Using the equation,

TME i + W ext = TME f 312 500 J + (-8000 • d) = 0 J

Using some algebra it can be shown that d=39.1 m

PE = 0 J (the car's height is zero) KE = 0.5*6000*(20)^2 = 1 200 000 J

PE = 0 J (the car's height is zero) KE = 0.5*6000*(5)^2 = 75 000 J

The work done is F • 20 • cos 180 = -20•F

TME i + W ext = TME f 1 200 000 J + (-20*F) = 75 000 J

Using some algebra, it can be shown that 20*F = 1 125 000 and so F = 56 250 N

The question pertains to the can of peaches; so focus on the can (not the cart).

PE = 0.25 kg * 9.8 m/s/s * 2 m = 4.9 J KE = 0 J (the peach can is at rest)

PE = 0 J (the can's height is zero) KE = 0 J (the peach can is at rest)

The work done is 500 N*d*cos 180 = -500*d

TME i + W ext = TME f 4.9 J + (-500*d) = 0 J

Using some algebra, it can be shown that d = 0.0098 m (9.8 mm)

Stopping Distance

All three of the above problems have one thing in common: there is a force that does work over a distance in order to remove mechanical energy from an object. The force acts opposite the object's motion (angle between force and displacement is 180 degrees) and thus does negative work. Negative work results in a loss of the object's total amount of mechanical energy . In each situation, the work is related to the kinetic energy change. And since the distance (d) over which the force does work is related to the work and since the velocity squared (v^2) of the object is related to the kinetic energy, there must also be a direct relation between the stopping distance and the velocity squared. Observe the derivation below.

KE i + W ext = 0 J

0.5•m•v i 2 + F•d•cos(Theta) = 0 J

0.5•m•v i 2 = F•d

The above equation depicts stopping distance as being dependent upon the square of the velocity . This means that a twofold increase in velocity would result in a fourfold (two squared) increase in stopping distance. A threefold increase in velocity would result in a nine-fold (three squared) increase in stopping distance. And a fourfold increase in velocity would result in a sixteen-fold (four squared) increase in stopping distance. This is one more example in which an equation becomes more than a mere algebraic recipe for solving problems. Equations can also be powerful guides to thinking about how two quantities are related to each other. In the case of a horizontal force bringing an object to a stop over some horizontal distance, the stopping distance of the object is related to the square of the velocity of the object.

The above principle - that stopping distance is proportional to velocity squared - is often the focus of a popular physics lab. A Hot wheels car is rolled down an inclined plane to the floor below. Once reaching the floor, it strikes a computer diskette box and skids to a stop as a result of the friction between the car/box system and the floor. A photo gate time is used to determine the speed of the car prior to striking the box. Several trials are performed and a data set is collected and plotted. As the speed of the car is increased, the stopping distance is increased. If the data are plotted, then a clear power relationship is seen. If power regression is performed on the data set, the results tend to show that d = k•v 2 where k is a constant of proportionality.

The examples mentioned on this page involve the application of the work-energy relationship to situations involving external or nonconservative forces doing work. An entirely different outcome results in situations in which there is no work done by external forces. The next part of Lesson 2 involves an analysis of these situations.

Five-star Bayern roll over Rostov

Tuesday, September 13, 2016

Article summary

Robert Lewandowski's penalty was added to by Thomas Müller, Juan Bernat and two Joshua Kimmich strikes as Bayern won 5-0 to give Russian newcomers Rostov a harsh introduction.

Article top media content

Article body.

Bayern München produced a five-star display to dispatch group stage debutants FC Rostov, setting a new UEFA Champions League record with a 13th successive home win.

• Barcelona and Bayern begin in style
• Also in Group D: PSV 0-1 Atlético

Rostov did not help their own cause by giving away two soft goals in the first half, the first a Robert Lewandowski penalty and the second on the stroke of half-time to Thomas Müller, on his 27th birthday.

Bayern had hardly broken sweat in the first 45 minutes, but began to press harder in the second. The impressive Joshua Kimmich scored twice in quick succession before Juan Bernat added a fifth in stoppage time.

Key Player: Joshua Kimmich (Bayern) When Ancelotti announced that Joshua Kimmich would be in the starting XI, many assumed he would replace Philipp Lahm at right-back. Instead, the Italian put him in the heart of his midfield and the 21-year-old didn't disappoint. Not disheartened by two failed attempts on goal in the first half, the Bayern youngster continued to push forward to exploit the space afforded him and was rewarded with his first UEFA Champions League goals.

Bayern's depth Being able to rest key players like Philipp Lahm, Franck Ribéry and Xabi Alonso for a UEFA Champions League match is not a luxury many teams enjoy, but Bayern have quality in abundance. Kimmich has clearly given Carlo Ancelotti something to think about, while the Italian still has to fit Jérôme Boateng into a defence that is yet to concede this season. Kingsley Coman and Arjen Robben are also yet to return to bolster an already devastating attack. 

Rostov's rude awakening Rostov discovered just how big the gulf in class is in the UEFA Champions League, as they were outplayed in Munich. Naivety as much as anything cost the Russian side as they gave away an unnecessary penalty, which was converted by the prolific Robert Lewandowski, but the sucker punch was to come on the stroke of half time when poor defending presented Thomas Müller with a simple finish to effectively end the contest.

UEFA.com team reporters' views

Jordan Maciel, Bayern ( @UEFAcomJordanM ) A good start to the campaign for Bayern, who never really had to get out of first gear. Rostov gave a good account of themselves in the first half and will rue conceding the opener from a penalty and then again so close to half-time. Ancelotti will have no complaints as his side claimed all three points and a healthy goal difference ahead of another meeting with Atlético.

Richard van Poortvliet, Rostov ( @UEFAcomRichVP ) Rostov offered little in attack, which is understandable given the size of the task of playing against one of the best sides in Europe. However, it's imperative the side from the south of Russia become more streetwise in their remaining games if they are to have any hope of qualifying.

Match reaction

Carlo Ancelotti, Bayern coach It was a good start to our Champions League campaign. It was difficult to score early on, we didn't have many chances. We got there. At the moment the team is in good condition, both physically and mentally.

Ivan Danilyants, Rostov coach We have never played against a team that played like Bayern today. We tried hard to hold them off and stay organised then the first goal came from an unfortunate penalty. The rest from quick counters. This side is different to the one under Pep Guardiola: they are much more direct.